Question

Draw a rough sketch of the curve \[y = \frac{x}{\pi} + 2 \sin^2 x\] and find the area between the x-axis, the curve and the ordinates x = 0 and x = π.

Answer 1

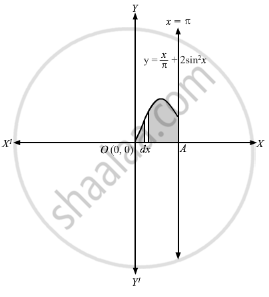
The table for  different values of x and y is 

X	0	`pi/6`	`pi/2`	`(5pi)/6`	`pi`
sin x	0	`1/2`	1	`1/2`	0
\[y = \frac{\pi}{2} + 2 \sin^2 x\]	0	`2/3`	`5/2`	`4/3`	1

\[y = \frac{x}{\pi} + 2 \sin^2 x ,\text{ is an arc cutting }y -\text{ axis at O(0, 0) and cutting }x = \pi \text{ at } \left( \pi, 1 \right)\]

\[\text{ Consider a vertical strip of length }= \left| y \right| \text{ and width }= dx \text{ in the first quadrant }\]

\[ \therefore\text{ Area of approximating rectangle }= \left| y \right| dx\]

\[\text{ The approximating rectangle moves from }x = 0\text{ to }x = \pi\]

\[ \Rightarrow\text{ Area of the shaded area }= \int_0^\pi \left| y \right| dx\]

\[ \Rightarrow A = \int_0^\pi y dx ..............\left\{ As, y > 0 \Rightarrow \left| y \right| = y \right\}\]

\[ \Rightarrow A = \int_0^\pi \left( \frac{x}{\pi} + 2 \sin^2 x \right) dx\]

\[ \Rightarrow A = \frac{1}{\pi} \int_0^\pi x dx + 2 \int_0^\pi \sin^2 x dx\]

\[ \Rightarrow A = \frac{1}{\pi} \left[ \frac{x^2}{2} \right]_0^\pi + 2 \left[ \frac{x}{2} - \frac{1}{2}\sin x \cos x \right]_0^\pi \]

\[ \Rightarrow A = \frac{\pi^2}{2\pi} + \frac{2}{2}\left[ \pi - \frac{1}{2}\sin \pi \cos \pi - 0 \right]\]

\[ \Rightarrow A = \frac{\pi}{2} + \pi\]

\[ \Rightarrow A = \frac{3\pi}{2}\text{ sq . units }\]

\[ \therefore\text{ Area of the curve enclosed between }x = 0\text{ and }x = \pi\text{ is }\frac{3\pi}{2}\text{ sq . units }\]