Advertisements
Advertisements
प्रश्न
If An be the area bounded by the curve y = (tan x)n and the lines x = 0, y = 0 and x = π/4, then for x > 2
विकल्प
An + An −2 = \[\frac{1}{n - 1}\]
An + An − 2 < \[\frac{1}{n - 1}\]
An − An − 2 = \[\frac{1}{n - 1}\]
none of these
उत्तर
An + An −2 =\[\frac{1}{n - 1}\]
\[A_n =\] Area bounded by the curve "
\[y = \left\{ \tan\left( x \right) \right\}^n = \tan^n \left( x \right)\] and the lines \[x = 0, y = 0,\] and \[x = \frac{\pi}{4}\]
Therefore,
\[A_n = \int_0^\frac{\pi}{4} \tan^n \left( x \right) d x\]
\[ \Rightarrow A_{n - 2} = \int_0^\frac{\pi}{4} \tan^{n - 2} \left( x \right) d x\]
Consider, "
\[A_n = \int_0^\frac{\pi}{4} \tan^n \left( x \right) d x\]
\[\Rightarrow A_n = \int_0^\frac{\pi}{4} \left\{ \tan^{n - 2} \left( x \right) \right\}\left\{ \tan^2 \left( x \right) \right\} d x\]
\[ \Rightarrow A_n = \int_0^\frac{\pi}{4} \left\{ \tan^{n - 2} \left( x \right) \right\}\left\{ \sec^2 \left( x \right) - 1 \right\} d x\]
\[ \Rightarrow A_n = \int_0^\frac{\pi}{4} \left\{ \tan^{n - 2} \left( x \right) \sec^2 \left( x \right) - \tan^{n - 2} \left( x \right) \right\} d x\]
\[ \Rightarrow A_n = \int_0^\frac{\pi}{4} \left\{ \tan^{n - 2} \left( x \right) \sec^2 \left( x \right) \right\} d x - \int_0^\frac{\pi}{4} \tan^{n - 2} \left( x \right) d x\]
Now,
\[\text{Let }u = \tan\left( x \right)\]
\[ \Rightarrow d u = \sec^2 \left( x \right)dx\]
Also, when \[x = 0, u = 0\] and when \[x = \frac{\pi}{4}, u = 1\]
Therefore,
\[= \int_0^1 \left( u^{n - 2} \right) d u\]
\[ = \left[ \frac{u^{n - 1}}{n - 1} \right]_0^1 \]
\[ = \left[ \frac{1}{n - 1} - 0 \right] = \frac{1}{n - 1}\]
APPEARS IN
संबंधित प्रश्न
Using integration, find the area of the region bounded by the lines y = 2 + x, y = 2 – x and x = 2.
Draw a rough sketch of the curve and find the area of the region bounded by curve y2 = 8x and the line x =2.
Find the area of the minor segment of the circle \[x^2 + y^2 = a^2\] cut off by the line \[x = \frac{a}{2}\]
Draw a rough sketch of the region {(x, y) : y2 ≤ 3x, 3x2 + 3y2 ≤ 16} and find the area enclosed by the region using method of integration.
Find the area, lying above x-axis and included between the circle x2 + y2 = 8x and the parabola y2 = 4x.
Find the area of the region in the first quadrant enclosed by x-axis, the line y = \[\sqrt{3}x\] and the circle x2 + y2 = 16.
Find the area of the region bounded by y = | x − 1 | and y = 1.
If the area bounded by the parabola \[y^2 = 4ax\] and the line y = mx is \[\frac{a^2}{12}\] sq. units, then using integration, find the value of m.
If the area enclosed by the parabolas y2 = 16ax and x2 = 16ay, a > 0 is \[\frac{1024}{3}\] square units, find the value of a.
Find the area bounded by the parabola y2 = 4x and the line y = 2x − 4 By using horizontal strips.
Find the area of the region bounded by the parabola y2 = 2x and the straight line x − y = 4.
The area included between the parabolas y2 = 4x and x2 = 4y is (in square units)
The area of the region formed by x2 + y2 − 6x − 4y + 12 ≤ 0, y ≤ x and x ≤ 5/2 is ______ .
The area bounded by the parabola y2 = 4ax, latusrectum and x-axis is ___________ .
Area bounded by parabola y2 = x and straight line 2y = x is _________ .
The area bounded by the curve y = f (x), x-axis, and the ordinates x = 1 and x = b is (b −1) sin (3b + 4). Then, f (x) is __________ .
Smaller area enclosed by the circle x2 + y2 = 4 and the line x + y = 2 is
Area of the region bounded by the curve y2 = 4x, y-axis and the line y = 3, is
Find the coordinates of a point of the parabola y = x2 + 7x + 2 which is closest to the straight line y = 3x − 3.
Find the area bounded by the parabola y2 = 4x and the line y = 2x − 4 By using vertical strips.
Sketch the graphs of the curves y2 = x and y2 = 4 – 3x and find the area enclosed between them.
Find the area of the region above the x-axis, included between the parabola y2 = ax and the circle x2 + y2 = 2ax.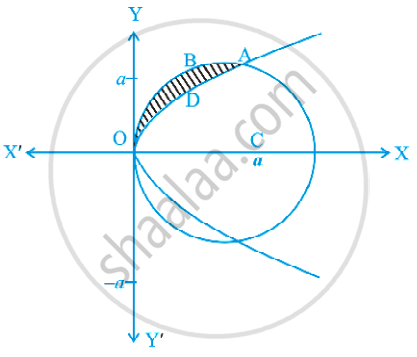
The area of the region bounded by the curve y = x2 and the line y = 16 ______.
The area of the region bounded by the curve x = y2, y-axis and the line y = 3 and y = 4 is ______.
Find the area of the region bounded by the curves y2 = 9x, y = 3x
Find the area of the region enclosed by the parabola x2 = y and the line y = x + 2
Find the area enclosed by the curve y = –x2 and the straight lilne x + y + 2 = 0
Find the area bounded by the lines y = 4x + 5, y = 5 – x and 4y = x + 5.
Find the area bounded by the curve y = 2cosx and the x-axis from x = 0 to x = 2π
The area of the region bounded by the curve y = `sqrt(16 - x^2)` and x-axis is ______.
Area of the region bounded by the curve y = cosx between x = 0 and x = π is ______.
The area of the region bounded by parabola y2 = x and the straight line 2y = x is ______.
The area of the region bounded by the curve y = x + 1 and the lines x = 2 and x = 3 is ______.
Using integration, find the area of the region bounded between the line x = 4 and the parabola y2 = 16x.
The area bounded by the curve `y = x^3`, the `x`-axis and ordinates `x` = – 2 and `x` = 1
The area bounded by the curve `y = x|x|`, `x`-axis and the ordinate `x` = – 1 and `x` = 1 is given by
Make a rough sketch of the region {(x, y): 0 ≤ y ≤ x2, 0 ≤ y ≤ x, 0 ≤ x ≤ 2} and find the area of the region using integration.
Sketch the region enclosed bounded by the curve, y = x |x| and the ordinates x = −1 and x = 1.
Hence find the area bounded by the curve, y = x |x| and the coordinates x = −1 and x = 1.
