Advertisements
Advertisements
प्रश्न
Find the area enclosed by the curve y = –x2 and the straight lilne x + y + 2 = 0
उत्तर
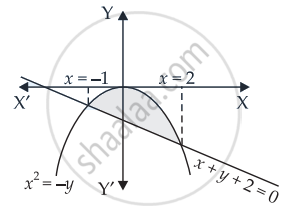
We are given that y = –x2 or x2 = –y
And the line x + y + 2 = 0
Solving the two equations,
We get x – x2 + 2 = 0
⇒ x2 – x – 2 = 0
⇒ x2 – 2x + x – 2 = 0
⇒ x(x – 2) + 1(x – 2) = 0
⇒ (x – 2)(x + 1) = 0
∴ x = –1, 2
Area of the required shaded region
= `|int_-1^2 (-x - 2) "d"x - int_-1^2 - x^2 "d"x|`
⇒ `|-[x^2/2 + x]_-1^2 + 1/3 [x^3]_-1^2|`
⇒ `|-[4/2 + 4) - (1/2 - 2)] + 1/3(8 + 1)|`
⇒ `|-(6 + 3/2) + 1/3(9)|`
⇒ `|- 15/2 + 3|`
⇒ `|(-15 + 6)/2| = |(-9)/2|`
= `9/2` sq.units
APPEARS IN
संबंधित प्रश्न
Find the area lying above the x-axis and under the parabola y = 4x − x2.
Draw a rough sketch of the graph of the function y = 2 \[\sqrt{1 - x^2}\] , x ∈ [0, 1] and evaluate the area enclosed between the curve and the x-axis.
Determine the area under the curve y = \[\sqrt{a^2 - x^2}\] included between the lines x = 0 and x = a.
Using definite integrals, find the area of the circle x2 + y2 = a2.
Using integration, find the area of the region bounded by the following curves, after making a rough sketch: y = 1 + | x + 1 |, x = −2, x = 3, y = 0.
Draw a rough sketch of the curve y = \[\frac{\pi}{2} + 2 \sin^2 x\] and find the area between x-axis, the curve and the ordinates x = 0, x = π.
Compare the areas under the curves y = cos2 x and y = sin2 x between x = 0 and x = π.
Find the area of the region bounded by the curve \[x = a t^2 , y = 2\text{ at }\]between the ordinates corresponding t = 1 and t = 2.
Find the area of the region \[\left\{ \left( x, y \right): \frac{x^2}{a^2} + \frac{y^2}{b^2} \leq 1 \leq \frac{x}{a} + \frac{y}{b} \right\}\]
Sketch the region bounded by the curves y = x2 + 2, y = x, x = 0 and x = 1. Also, find the area of this region.
Find the area of the region bounded by y = | x − 1 | and y = 1.
Find the area of the region enclosed by the parabola x2 = y and the line y = x + 2.
Find the area of the region enclosed between the two curves x2 + y2 = 9 and (x − 3)2 + y2 = 9.
Find the area of the region between the parabola x = 4y − y2 and the line x = 2y − 3.
The area bounded by y = 2 − x2 and x + y = 0 is _________ .
The area bounded by the curve y = 4x − x2 and the x-axis is __________ .
The area bounded by the curve y = x |x| and the ordinates x = −1 and x = 1 is given by
Find the area of the region bound by the curves y = 6x – x2 and y = x2 – 2x
Find the area of the region bounded by the curve y = x3 and y = x + 6 and x = 0
Find the area of the region bounded by y = `sqrt(x)` and y = x.
Draw a rough sketch of the region {(x, y) : y2 ≤ 6ax and x 2 + y2 ≤ 16a2}. Also find the area of the region sketched using method of integration.
Using integration, find the area of the region in the first quadrant enclosed by the line x + y = 2, the parabola y2 = x and the x-axis.
If a and c are positive real numbers and the ellipse `x^2/(4c^2) + y^2/c^2` = 1 has four distinct points in common with the circle `x^2 + y^2 = 9a^2`, then
Find the area of the region bounded by `x^2 = 4y, y = 2, y = 4`, and the `y`-axis in the first quadrant.
Find the area of the region bounded by the curve `y = x^2 + 2, y = x, x = 0` and `x = 3`
The area bounded by the curve `y = x^3`, the `x`-axis and ordinates `x` = – 2 and `x` = 1
Find the area of the region bounded by curve 4x2 = y and the line y = 8x + 12, using integration.
For real number a, b (a > b > 0),
let Area `{(x, y): x^2 + y^2 ≤ a^2 and x^2/a^2 + y^2/b^2 ≥ 1}` = 30π
Area `{(x, y): x^2 + y^2 ≥ b^2 and x^2/a^2 + y^2/b^2 ≤ 1}` = 18π.
Then the value of (a – b)2 is equal to ______.
Evaluate:
`int_0^1x^2dx`
