Advertisements
Advertisements
प्रश्न
Find the area of the region bounded by curve 4x2 = y and the line y = 8x + 12, using integration.
उत्तर
Given curve is 4x2 = y and line is y = 8x + 12
On solving both equations, we get
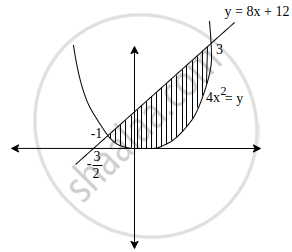
4x2 = 8x + 12
⇒ x2 = 2x + 3
⇒ x2 – 2x – 3 = 0
⇒ x = 3, –1
Required area = `int_-1^3 {(8x + 12) - 4x^2}dx`
= `4int_-1^3 (2x + 3 - x^2)dx`
= `4[x^2 + 3x - x^3/3]_-1^3`
= `4[(9 + 9 - 9) - (1 - 3 + 1/3)]`
= `4(9 + 2 - 1/3)`
= `4(11 - 1/3)`
= `4 xx 32/2`
= `128/2` sq.units
APPEARS IN
संबंधित प्रश्न
Find the area of the region common to the circle x2 + y2 =9 and the parabola y2 =8x
Using the method of integration, find the area of the triangular region whose vertices are (2, -2), (4, 3) and (1, 2).
Area bounded by the curve y = x3, the x-axis and the ordinates x = –2 and x = 1 is ______.
The area bounded by the curve y = x | x|, x-axis and the ordinates x = –1 and x = 1 is given by ______.
[Hint: y = x2 if x > 0 and y = –x2 if x < 0]
Find the equation of a curve passing through the point (0, 2), given that the sum of the coordinates of any point on the curve exceeds the slope of the tangent to the curve at that point by 5.
Draw a rough sketch of the curve and find the area of the region bounded by curve y2 = 8x and the line x =2.
Using integration, find the area of the region bounded by the following curves, after making a rough sketch: y = 1 + | x + 1 |, x = −2, x = 3, y = 0.
Sketch the graph y = | x + 1 |. Evaluate\[\int\limits_{- 4}^2 \left| x + 1 \right| dx\]. What does the value of this integral represent on the graph?
Find the area bounded by the ellipse \[\frac{x^2}{a^2} + \frac{y^2}{b^2} = 1\] and the ordinates x = ae and x = 0, where b2 = a2 (1 − e2) and e < 1.
Find the area of the region in the first quadrant bounded by the parabola y = 4x2 and the lines x = 0, y = 1 and y = 4.
Calculate the area of the region bounded by the parabolas y2 = x and x2 = y.
Draw a rough sketch of the region {(x, y) : y2 ≤ 3x, 3x2 + 3y2 ≤ 16} and find the area enclosed by the region using method of integration.
Sketch the region bounded by the curves y = x2 + 2, y = x, x = 0 and x = 1. Also, find the area of this region.
Find the area of the region in the first quadrant enclosed by the x-axis, the line y = x and the circle x2 + y2= 32.
Find the area of the circle x2 + y2 = 16 which is exterior to the parabola y2 = 6x.
Find the area of the region bounded by the curve y = \[\sqrt{1 - x^2}\], line y = x and the positive x-axis.
If the area enclosed by the parabolas y2 = 16ax and x2 = 16ay, a > 0 is \[\frac{1024}{3}\] square units, find the value of a.
Find the area bounded by the parabola x = 8 + 2y − y2; the y-axis and the lines y = −1 and y = 3.
Area bounded by the curve y = x3, the x-axis and the ordinates x = −2 and x = 1 is ______.
The area of the circle x2 + y2 = 16 enterior to the parabola y2 = 6x is
Sketch the graphs of the curves y2 = x and y2 = 4 – 3x and find the area enclosed between them.
The area enclosed by the circle x2 + y2 = 2 is equal to ______.
Find the area of the region bounded by the curves y2 = 9x, y = 3x
Find the area bounded by the curve y = sinx between x = 0 and x = 2π.
Find the area bounded by the curve y = 2cosx and the x-axis from x = 0 to x = 2π
The area of the region bounded by the curve y = `sqrt(16 - x^2)` and x-axis is ______.
Find the area of the region bounded by the curve `y^2 - x` and the line `x` = 1, `x` = 4 and the `x`-axis.
Let f : [–2, 3] `rightarrow` [0, ∞) be a continuous function such that f(1 – x) = f(x) for all x ∈ [–2, 3]. If R1 is the numerical value of the area of the region bounded by y = f(x), x = –2, x = 3 and the axis of x and R2 = `int_-2^3 xf(x)dx`, then ______.
Make a rough sketch of the region {(x, y) : 0 ≤ y ≤ x2 + 1, 0 ≤ y ≤ x + 1, 0 ≤ x ≤ 2} and find the area of the region, using the method of integration.
Sketch the region enclosed bounded by the curve, y = x |x| and the ordinates x = −1 and x = 1.
