Advertisements
Advertisements
प्रश्न
Area of the region in the first quadrant enclosed by the x-axis, the line y = x and the circle x2 + y2 = 32 is ______.
विकल्प
16π sq.units
4π sq.units
32π sq.units
24 sq.units
उत्तर
Area of the region in the first quadrant enclosed by the x-axis, the line y = x and the circle x2 + y2 = 32 is 4π sq.units.
Explanation:
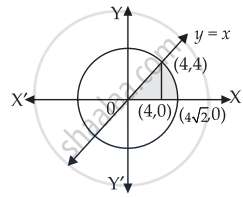
Given equation of circle is x2 + y2 = 32
⇒ x2 + y2 = `(4sqrt(2))^2` and the line is y = x and the x-axis.
Solving the two equations
We have x2 + x2 = 32
⇒ 2x2 = 32
⇒ x2 = 16
∴ x = ± 4
Required area = `int_0^4 x "d"x + int_4^(4sqrt(2)) sqrt((4sqrt(2))^2 - x^2) "d"x`
= `1/2 [x^2]_0^4 + [x/2 sqrt((4sqrt(2))^2 - x^2) + 32/2 sin^-1 x/(4sqrt(2))]_4^(4/sqrt(2))`
= `1/2 [16 - 0] + [0 + 16 sin^-1 ((4sqrt(2))/(4sqrt(2))) - 2sqrt(32 - 16) - 16sin^-1 4/(4sqrt(2))]`
= `8 + [16 sin^-1 (1) - 8 - 16sin^-1 1/sqrt(2)]`
= `8 + 16 * pi/2 - 8 - 16 * pi/4`
= `8pi - 4pi`
= 4π sq.units
APPEARS IN
संबंधित प्रश्न
Using the method of integration, find the area of the triangular region whose vertices are (2, -2), (4, 3) and (1, 2).
Find the area lying above the x-axis and under the parabola y = 4x − x2.
Using definite integrals, find the area of the circle x2 + y2 = a2.
Find the area of the minor segment of the circle \[x^2 + y^2 = a^2\] cut off by the line \[x = \frac{a}{2}\]
Find the area of the region common to the circle x2 + y2 = 16 and the parabola y2 = 6x.
Find the area enclosed by the parabolas y = 5x2 and y = 2x2 + 9.
Find the area bounded by the parabola y = 2 − x2 and the straight line y + x = 0.
Find the area enclosed by the curves 3x2 + 5y = 32 and y = | x − 2 |.
If the area enclosed by the parabolas y2 = 16ax and x2 = 16ay, a > 0 is \[\frac{1024}{3}\] square units, find the value of a.
Find the area bounded by the parabola y2 = 4x and the line y = 2x − 4 By using horizontal strips.
The area included between the parabolas y2 = 4x and x2 = 4y is (in square units)
Area bounded by parabola y2 = x and straight line 2y = x is _________ .
The area of the circle x2 + y2 = 16 enterior to the parabola y2 = 6x is
Draw a rough sketch of the curve y2 = 4x and find the area of region enclosed by the curve and the line y = x.
Find the area of the region bounded by the parabolas y2 = 6x and x2 = 6y.
Find the area of the region bounded by the curve y2 = 4x, x2 = 4y.
Find the area bounded by the curve y = sinx between x = 0 and x = 2π.
Draw a rough sketch of the region {(x, y) : y2 ≤ 6ax and x 2 + y2 ≤ 16a2}. Also find the area of the region sketched using method of integration.
Draw a rough sketch of the given curve y = 1 + |x +1|, x = –3, x = 3, y = 0 and find the area of the region bounded by them, using integration.
Using integration, find the area of the region `{(x, y): 0 ≤ y ≤ sqrt(3)x, x^2 + y^2 ≤ 4}`
The region bounded by the curves `x = 1/2, x = 2, y = log x` and `y = 2^x`, then the area of this region, is
Area lying in the first quadrant and bounded by the circle `x^2 + y^2 = 4` and the lines `x + 0` and `x = 2`.
Make a rough sketch of the region {(x, y): 0 ≤ y ≤ x2, 0 ≤ y ≤ x, 0 ≤ x ≤ 2} and find the area of the region using integration.
The area of the region S = {(x, y): 3x2 ≤ 4y ≤ 6x + 24} is ______.
Let g(x) = cosx2, f(x) = `sqrt(x)`, and α, β (α < β) be the roots of the quadratic equation 18x2 – 9πx + π2 = 0. Then the area (in sq. units) bounded by the curve y = (gof)(x) and the lines x = α, x = β and y = 0, is ______.
The area of the region bounded by the parabola (y – 2)2 = (x – 1), the tangent to it at the point whose ordinate is 3 and the x-axis is ______.
Find the area of the smaller region bounded by the curves `x^2/25 + y^2/16` = 1 and `x/5 + y/4` = 1, using integration.
Using integration, find the area of the region bounded by y = mx (m > 0), x = 1, x = 2 and the X-axis.
Hence find the area bounded by the curve, y = x |x| and the coordinates x = −1 and x = 1.
