Advertisements
Advertisements
प्रश्न
Using integration, find the area of the region bounded by y = mx (m > 0), x = 1, x = 2 and the X-axis.
उत्तर
Area = `int_1^2 mx dx`

= `m[x^2/2]_1^2`
= `m/2 [4 - 1]`
= `(3m)/2` sq. units.
APPEARS IN
संबंधित प्रश्न
triangle bounded by the lines y = 0, y = x and x = 4 is revolved about the X-axis. Find the volume of the solid of revolution.
Find the area of the region common to the circle x2 + y2 =9 and the parabola y2 =8x
Find the area of the sector of a circle bounded by the circle x2 + y2 = 16 and the line y = x in the ftrst quadrant.
Find the area of the region bounded by the curve xy − 3x − 2y − 10 = 0, x-axis and the lines x = 3, x = 4.
Show that the areas under the curves y = sin x and y = sin 2x between x = 0 and x =\[\frac{\pi}{3}\] are in the ratio 2 : 3.
Find the area enclosed by the curve x = 3cost, y = 2sin t.
Find the area of the region {(x, y) : y2 ≤ 8x, x2 + y2 ≤ 9}.
Find the area, lying above x-axis and included between the circle x2 + y2 = 8x and the parabola y2 = 4x.
Prove that the area common to the two parabolas y = 2x2 and y = x2 + 4 is \[\frac{32}{3}\] sq. units.
Find the area of the region between the parabola x = 4y − y2 and the line x = 2y − 3.
Find the area of the region bounded by the parabola y2 = 2x and the straight line x − y = 4.
The area bounded by the curve y = loge x and x-axis and the straight line x = e is ___________ .
The area bounded by the curve y = x4 − 2x3 + x2 + 3 with x-axis and ordinates corresponding to the minima of y is _________ .
Area bounded by parabola y2 = x and straight line 2y = x is _________ .
The area bounded by the y-axis, y = cos x and y = sin x when 0 ≤ x ≤ \[\frac{\pi}{2}\] is _________ .
Find the area of the region bounded by the parabolas y2 = 6x and x2 = 6y.
Find the area of the region above the x-axis, included between the parabola y2 = ax and the circle x2 + y2 = 2ax.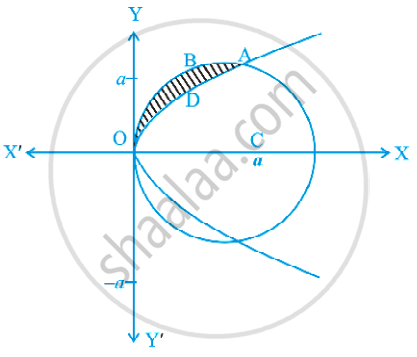
Find the area enclosed by the curve y = –x2 and the straight lilne x + y + 2 = 0
Find the area of the region bounded by the curve y2 = 2x and x2 + y2 = 4x.
Draw a rough sketch of the given curve y = 1 + |x +1|, x = –3, x = 3, y = 0 and find the area of the region bounded by them, using integration.
The area of the region bounded by the curve x2 = 4y and the straight line x = 4y – 2 is ______.
Area of the region in the first quadrant enclosed by the x-axis, the line y = x and the circle x2 + y2 = 32 is ______.
The area of the region bounded by the curve x = 2y + 3 and the y lines. y = 1 and y = –1 is ______.
If a and c are positive real numbers and the ellipse `x^2/(4c^2) + y^2/c^2` = 1 has four distinct points in common with the circle `x^2 + y^2 = 9a^2`, then
Area of the region bounded by the curve y = |x + 1| + 1, x = –3, x = 3 and y = 0 is
Area (in sq.units) of the region outside `|x|/2 + |y|/3` = 1 and inside the ellipse `x^2/4 + y^2/9` = 1 is ______.
Using integration, find the area of the region bounded by the curve y2 = 4x and x2 = 4y.
Sketch the region enclosed bounded by the curve, y = x |x| and the ordinates x = −1 and x = 1.
