Question

The area bounded by the y-axis, y = cos x and y = sin x when 0 ≤ x ≤ \[\frac{\pi}{2}\] is _________ .

विकल्प

• 2\[\left( \sqrt{2} - 1 \right)\]

• \[\sqrt{2} - 1\]
• \[\sqrt{2} + 1\]
• \[\sqrt{2}\]

Answer 1

\[\sqrt{2} - 1\]

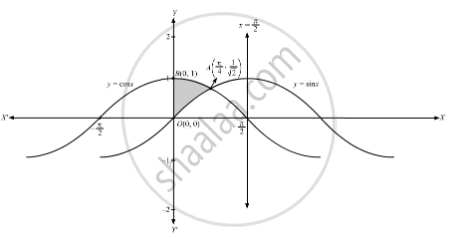
Points of intersection is obtained by solving
\[y = \sin x\text{ and }y = \cos x \]
\[ \therefore \sin x = \cos x\]
\[ \Rightarrow x = \frac{\pi}{4}\]
\[\text{ Thus the two functions intersect at }x = \frac{\pi}{4}\]
\[ \Rightarrow y = \sin \frac{\pi}{4} = \frac{1}{\sqrt{2}}\]
\[\text{ Hence A }\left( \frac{\pi}{4}, \frac{1}{\sqrt{2}} \right)\text{ is the point of intersection.} \]
\[ \therefore\text{ Area bound by the curves and the }y - \text{ axis when }0 \leq x \leq \frac{\pi}{2}, \]
\[A = \int_0^\frac{1}{\sqrt{2}} \left| x_1 \right| dy + \int_\frac{1}{\sqrt{2}}^1 \left| x_2 \right| dy\]
\[ = \int_0^\frac{1}{\sqrt{2}} x_1 dy + \int_\frac{1}{\sqrt{2}}^1 x_2 dy\]
\[ = \int_0^\frac{1}{\sqrt{2}} \sin^{- 1} y dy + \int_\frac{1}{\sqrt{2}}^1 \cos^{- 1} y dy\]
\[ = \left[ y \sin^{- 1} y + \sqrt{1 - y^2} \right]_0^\frac{1}{\sqrt{2}} + \left[ y \cos {}^{- 1} y - \sqrt{1 - y^2} \right]_\frac{1}{\sqrt{2}}^1 \]
\[ = \left[ \frac{1}{\sqrt{2}} \sin^{- 1} \frac{1}{\sqrt{2}} + \sqrt{1 - \frac{1}{2}} - 1 \right] + \left[ 1 \times \cos {}^{- 1} 1 - 0 - \frac{1}{\sqrt{2}}\cos {}^{- 1} \frac{1}{\sqrt{2}} + \sqrt{1 - \frac{1}{2}} \right]\]
\[ = \left[ \frac{1}{\sqrt{2}} \times \frac{\pi}{4} + \frac{1}{\sqrt{2}} - 1 \right] + \left[ 0 - \frac{1}{\sqrt{2}} \times \frac{\pi}{4} + \frac{1}{\sqrt{2}} \right]\]
\[ = \frac{1}{\sqrt{2}} + \frac{1}{\sqrt{2}} - 1\]
\[ = \frac{2}{\sqrt{2}} - 1\]
\[ = \left( \sqrt{2} - 1 \right)\text{ sq units }\]