Question

The area bounded by the parabola y² = 8x, the x-axis and the latusrectum is ___________ .

विकल्प

• \[\frac{16}{3}\]
• \[\frac{23}{3}\]
• \[\frac{32}{3}\]
• \[\frac{16\sqrt{2}}{3}\]

Answer 1

\[\frac{16}{3}\]

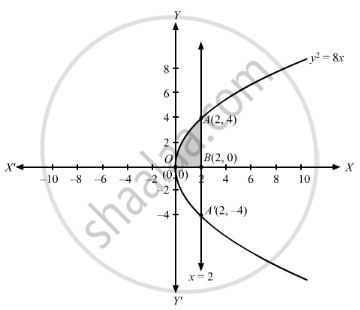
y² = 8x represents a parabola opening side ways , with vertex at O(0, 0) and Focus at B(2, 0)
Thus AA' represents the latus rectum of the parabola.
The points of intersection of the parabola and latus rectum are  A(2, 4)  and A'(2, −4)
Area bound by curve , x-axis and latus rectum  is the area OABO,
\[\text{ The approximating rectangle of width = dx and length }= \left| y \right| \text{ has area }= \left| y \right| dx,\text{ and moves from }x = 0\text{ to }x = 2\]
\[\text{ area }\left( OABO \right) = \int_0^2 \left| y \right| dx\]
\[ = \int_0^2 y dx ............\left\{ y > 0 , \Rightarrow \left| y \right| = y \right\}\]
\[ = \int_0^2 \sqrt{8x}dx\]
\[ = 2\sqrt{2} \int_0^2 \sqrt{x}dx\]
\[ = 2\sqrt{2} \left[ \frac{x^\frac{3}{2}}{\frac{3}{2}} \right]_0^2 \]
\[ = 2\sqrt{2} \times \frac{2}{3}\left( 2^\frac{3}{2} - 0 \right)\]
\[ = 4\frac{\sqrt{2}}{3} \times 2\sqrt{2}\]
\[ = \frac{16}{3} \text{ sq units }\]