Advertisements
Advertisements
प्रश्न
Compute the area bounded by the lines x + 2y = 2, y – x = 1 and 2x + y = 7.
उत्तर
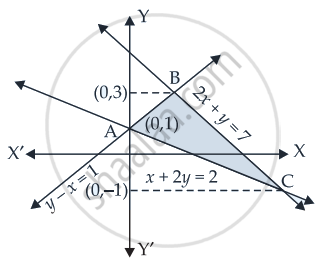
Given that: x + 2y = 2 .....(i)
y – x = 1 ......(ii)
And 2x + y = 7 ......(iii)
| x | 0 | 2 |
| y | 1 | 0 |
| x | 0 | –1 |
| y | 1 | 0 |
| x | 0 | `7/2` |
| y | 7 | 0 |
Solving equations (ii) and (iii)
We get y = 1 + x
∴ 2x + 1 + x = 7
3x = 6
⇒ x = 2
∴ y = 1 + 2
= 3
Coordinates of B = (2, 3)
Solving equations (i) and (iii)
We get x + 2y = 2
∴ x = 2 – 2y
2x + y = 7
2(2 – 2y) + y = 7
⇒ 4 – 4y + y = 7
⇒ –3y = 3
∴ y = –1 and x = 4
∴ Coordinates of C = (4, – 1) and coordinates of A = (0, 1).
Taking the limits on y-axis, we get
`int_(-1)^3 x_"BC" "d"y - int_(-1)^1 x_"AC" "d"y - int_1^3 x_"AB" "d"y`
= `int_(-1)^3 (7 - y)/2 "d"y - int_(-1)^1 (2 - 2y) "d"y - int_1^3 (y - 1) "d"y`
= `1/2 [7y - y^2/2]_-1^2 - 2[y - y^2/2]_-1^1 - [y^2/2 - y]_1^3`
= `1/2[(21 - 9/2) - (7 - 1/2)] - 2[(1 - 1/2) - (-1 - 1/2)] - [(9/2 - 3) - (1/2 - 1)]`
= `1/2[33/2 + 15/2] - 2[1/2 + 3/2] - [3/2 + 1/2]`
= `1/2 xx 24 - 2 xx 2 - 2`
⇒ 12 – 4 – 2 = 6 sq.units
Hence, the required area = 6 sq.units
APPEARS IN
संबंधित प्रश्न
Area bounded by the curve y = x3, the x-axis and the ordinates x = –2 and x = 1 is ______.
Find the area of ellipse `x^2/1 + y^2/4 = 1`
Draw a rough sketch to indicate the region bounded between the curve y2 = 4x and the line x = 3. Also, find the area of this region.
Make a rough sketch of the graph of the function y = 4 − x2, 0 ≤ x ≤ 2 and determine the area enclosed by the curve, the x-axis and the lines x = 0 and x = 2.
Draw a rough sketch of the graph of the function y = 2 \[\sqrt{1 - x^2}\] , x ∈ [0, 1] and evaluate the area enclosed between the curve and the x-axis.
Sketch the graph y = | x − 5 |. Evaluate \[\int\limits_0^1 \left| x - 5 \right| dx\]. What does this value of the integral represent on the graph.
Find the area of the region bounded by the curve xy − 3x − 2y − 10 = 0, x-axis and the lines x = 3, x = 4.
Draw a rough sketch of the curve \[y = \frac{x}{\pi} + 2 \sin^2 x\] and find the area between the x-axis, the curve and the ordinates x = 0 and x = π.
Find the area bounded by the ellipse \[\frac{x^2}{a^2} + \frac{y^2}{b^2} = 1\] and the ordinates x = ae and x = 0, where b2 = a2 (1 − e2) and e < 1.
Find the area of the region in the first quadrant bounded by the parabola y = 4x2 and the lines x = 0, y = 1 and y = 4.
Find the area of the region \[\left\{ \left( x, y \right): \frac{x^2}{a^2} + \frac{y^2}{b^2} \leq 1 \leq \frac{x}{a} + \frac{y}{b} \right\}\]
Find the area of the region {(x, y) : y2 ≤ 8x, x2 + y2 ≤ 9}.
Find the area of the region bounded by \[y = \sqrt{x}\] and y = x.
Find the area of the figure bounded by the curves y = | x − 1 | and y = 3 −| x |.
The closed area made by the parabola y = 2x2 and y = x2 + 4 is __________ .
Area bounded by parabola y2 = x and straight line 2y = x is _________ .
Find the area of the region bound by the curves y = 6x – x2 and y = x2 – 2x
The area of the region bounded by the curve x2 = 4y and the straight line x = 4y – 2 is ______.
The area of the region bounded by parabola y2 = x and the straight line 2y = x is ______.
The area of the region bounded by the curve y = sinx between the ordinates x = 0, x = `pi/2` and the x-axis is ______.
The curve x = t2 + t + 1,y = t2 – t + 1 represents
Find the area of the region bounded by the curve `y^2 - x` and the line `x` = 1, `x` = 4 and the `x`-axis.
Find the area of the region bounded by `x^2 = 4y, y = 2, y = 4`, and the `y`-axis in the first quadrant.
Smaller area bounded by the circle `x^2 + y^2 = 4` and the line `x + y = 2` is.
The area bounded by the curve `y = x^3`, the `x`-axis and ordinates `x` = – 2 and `x` = 1
The area (in sq.units) of the region A = {(x, y) ∈ R × R/0 ≤ x ≤ 3, 0 ≤ y ≤ 4, y ≤x2 + 3x} is ______.
Using integration, find the area of the region bounded by line y = `sqrt(3)x`, the curve y = `sqrt(4 - x^2)` and Y-axis in first quadrant.
Using integration, find the area bounded by the curve y2 = 4ax and the line x = a.
Evaluate:
`int_0^1x^2dx`
