Advertisements
Advertisements
प्रश्न
Draw a rough sketch to indicate the region bounded between the curve y2 = 4x and the line x = 3. Also, find the area of this region.
उत्तर
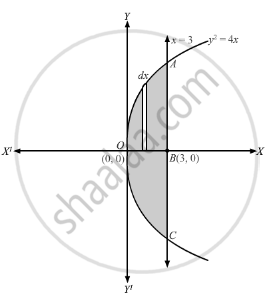
\[y^2 = 4x \text{ represents a parabola with vertex at (0, 0) and axis of symmetry along the + ve direction of } x\text{ axis }\]
\[x = 3\text{ is a line parallel to } y \text{ axis and cutting} x \text{ axis at (3, 0) }\]
\[\text{ Since }y^2 = 4x\text{ is symmetrical about }x\text{ axis , }\]
\[ \therefore\text{ Required area A }= \text{ OA }\hspace{0.167em} \text{CO }= 2 \times\text{ area OABO}\]
\[\text{ Slicing the area above }x \text{ axis into vertical strips of length }= \left| y \right|\text{ and width }= dx \]
\[\text{ Area of corresponding rectangle }= \left| y \right| dx\]
\[\text{ The corresponding rectangle moves from }x = 0\text{ to }x = 3\]
\[A = 2 \times\text{ area OABO }\]
\[ \Rightarrow A = 2 \int_0^3 \left| y \right| dx = 2 \int_0^3 \left| y \right| dx ..................\left[ As, \left| y \right| = y, y > 0 \right]\]
\[ \Rightarrow A = 2 \int_0^3 \sqrt{\left( 4x \right)} dx \]
\[ \Rightarrow A = 4 \int_0^3 \sqrt{x} dx \]
\[ \Rightarrow A = 4 \left[ \frac{x^\frac{3}{2}}{\frac{3}{2}} \right]_0^3 = \frac{8}{3} \left[ x^\frac{3}{2} \right]_0^3 = \frac{8}{3} \times 3\sqrt{3} = 8\sqrt{3}\text{ sq . units }\]
\[ \therefore \text{ Area of region bound by curve }y^2 = 4x \text{ and }x = 3\text{ is }8\sqrt{3}\text{ sq . units }\]
APPEARS IN
संबंधित प्रश्न
Find the area of the sector of a circle bounded by the circle x2 + y2 = 16 and the line y = x in the ftrst quadrant.
Find the area bounded by the curve y = sin x between x = 0 and x = 2π.
Using integration, find the area of the region bounded between the line x = 2 and the parabola y2 = 8x.
Draw the rough sketch of y2 + 1 = x, x ≤ 2. Find the area enclosed by the curve and the line x = 2.
Find the area of the region bounded by the curve xy − 3x − 2y − 10 = 0, x-axis and the lines x = 3, x = 4.
Draw a rough sketch of the region {(x, y) : y2 ≤ 3x, 3x2 + 3y2 ≤ 16} and find the area enclosed by the region using method of integration.
Prove that the area in the first quadrant enclosed by the x-axis, the line x = \[\sqrt{3}y\] and the circle x2 + y2 = 4 is π/3.
Find the area enclosed by the parabolas y = 5x2 and y = 2x2 + 9.
Using integration, find the area of the region bounded by the triangle whose vertices are (−1, 2), (1, 5) and (3, 4).
Find the area of the region bounded by the curve y = \[\sqrt{1 - x^2}\], line y = x and the positive x-axis.
If An be the area bounded by the curve y = (tan x)n and the lines x = 0, y = 0 and x = π/4, then for x > 2
The area of the region bounded by the parabola (y − 2)2 = x − 1, the tangent to it at the point with the ordinate 3 and the x-axis is _________ .
The area of the region \[\left\{ \left( x, y \right) : x^2 + y^2 \leq 1 \leq x + y \right\}\] is __________ .
The ratio of the areas between the curves y = cos x and y = cos 2x and x-axis from x = 0 to x = π/3 is ________ .
The area of the region (in square units) bounded by the curve x2 = 4y, line x = 2 and x-axis is
The area of the circle x2 + y2 = 16 enterior to the parabola y2 = 6x is
Draw a rough sketch of the curve y2 = 4x and find the area of region enclosed by the curve and the line y = x.
Find the equation of the parabola with latus-rectum joining points (4, 6) and (4, -2).
Find the area of the region bound by the curves y = 6x – x2 and y = x2 – 2x
Using integration, find the area of the region bounded by the parabola y2 = 4x and the circle 4x2 + 4y2 = 9.
Find the area of the curve y = sin x between 0 and π.
Find the area of the region bounded by the curve ay2 = x3, the y-axis and the lines y = a and y = 2a.
Find the area of the region above the x-axis, included between the parabola y2 = ax and the circle x2 + y2 = 2ax.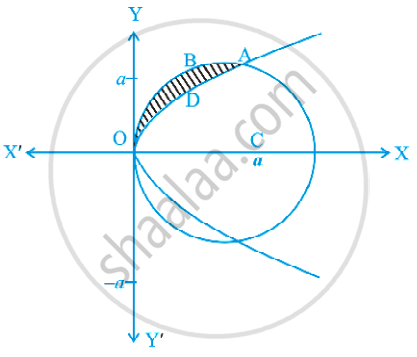
The area enclosed by the ellipse `x^2/"a"^2 + y^2/"b"^2` = 1 is equal to ______.
Find the area of region bounded by the line x = 2 and the parabola y2 = 8x
Using integration, find the area of the region `{(x, y): 0 ≤ y ≤ sqrt(3)x, x^2 + y^2 ≤ 4}`
Area lying in the first quadrant and bounded by the circle `x^2 + y^2 = 4` and the lines `x + 0` and `x = 2`.
Find the area of the region bounded by the curve `y^2 - x` and the line `x` = 1, `x` = 4 and the `x`-axis.
Find the area of the region bounded by `y^2 = 9x, x = 2, x = 4` and the `x`-axis in the first quadrant.
Find the area of the region bounded by `x^2 = 4y, y = 2, y = 4`, and the `y`-axis in the first quadrant.
The area bounded by the curve `y = x^3`, the `x`-axis and ordinates `x` = – 2 and `x` = 1
The area bounded by the curve `y = x|x|`, `x`-axis and the ordinate `x` = – 1 and `x` = 1 is given by
Find the area of the region bounded by curve 4x2 = y and the line y = 8x + 12, using integration.
Find the area of the region enclosed by the curves y2 = x, x = `1/4`, y = 0 and x = 1, using integration.
Area (in sq.units) of the region outside `|x|/2 + |y|/3` = 1 and inside the ellipse `x^2/4 + y^2/9` = 1 is ______.
Let g(x) = cosx2, f(x) = `sqrt(x)`, and α, β (α < β) be the roots of the quadratic equation 18x2 – 9πx + π2 = 0. Then the area (in sq. units) bounded by the curve y = (gof)(x) and the lines x = α, x = β and y = 0, is ______.
Evaluate:
`int_0^1x^2dx`
