SSC (English Medium)
SSC (Marathi Semi-English)
Academic Year: 2019-2020
Date: मार्च 2020
Duration: 2h
Advertisements
Select the appropriate alternative.
In ∆ABC and ∆PQR, in a one to one correspondence \[\frac{AB}{QR} = \frac{BC}{PR} = \frac{CA}{PQ}\]

∆PQR ~ ∆ABC
∆PQR ~ ∆CAB
∆CBA ~ ∆PQR
∆BCA ~ ∆PQR
Chapter: [0.01] Similarity
Some question and their alternative answer are given.
In a right-angled triangle, if sum of the squares of the sides making right angle is 169 then what is the length of the hypotenuse?
15
13
5
12
Chapter: [0.02] Pythagoras Theorem
Some question and their alternative answer are given.
In a right-angled triangle, if sum of the squares of the sides making right angle is 169 then what is the length of the hypotenuse?
15
13
5
12
Chapter: [0.02] Pythagoras Theorem
Choose the correct alternative answer for the following question.
1 cm3
0.001 cm3
0.0001 cm3
0.000001 cm3
Chapter: [0.07] Mensuration
Find the distance between the following pairs of point.
W `((- 7)/2 , 4)`, X (11, 4)
Chapter: [0.05] Co-ordinate Geometry
Prove that:
`(sin^2θ)/(cosθ) + cosθ = secθ`
Chapter: [0.06] Trigonometry
∆PQR ~ ∆LTR. In ∆PQR, PQ = 4.2 cm, QR = 5.4 cm, PR = 4.8 cm. Construct ∆PQR and ∆LTR, such that `"PQ"/"LT" = 3/4`.
Chapter: [0.04] Geometric Constructions [0.05] Co-ordinate Geometry
Seg RM and seg RN are tangent segments of a circle with centre O. Prove that seg OR bisects ∠MRN as well as ∠MON with the help of activity.
Chapter: [0.03] Circle
Advertisements
In the given figure, ∠QPR = 90°, seg PM ⊥ seg QR and Q–M–R, PM = 10, QM = 8, find QR.

Chapter: [0.02] Pythagoras Theorem
The dimensions of a cuboid are 44 cm, 21 cm, 12 cm. It is melted and a cone of height 24 cm is made. Find the radius of its base.
Chapter: [0.07] Mensuration
Prove that:
Sin4θ - cos4θ = 1 - 2cos2θ
Chapter: [0.06] Trigonometry
In adjoining figure, PQ ⊥ BC, AD ⊥ BC then find following ratios.

- `("A"(∆"PQB"))/("A"(∆"PBC"))`
- `("A"(∆"PBC"))/("A"(∆"ABC"))`
- `("A"(∆"ABC"))/("A"(∆"ADC"))`
- `("A"(∆"ADC"))/("A"(∆"PQC"))`
Chapter: [0.01] Similarity
In the given figure, M is the midpoint of QR. ∠PRQ = 90°. Prove that, PQ2 = 4PM2 – 3PR2.

Chapter: [0.02] Pythagoras Theorem
While landing at an airport, a pilot made an angle of depression of 20°. Average speed of the plane was 200 km/hr. The plane reached the ground after 54 seconds. Find the height at which the plane was when it started landing. (sin 20° = 0.342)
Chapter: [0.06] Trigonometry
From the top of a lighthouse, an observer looking at a ship makes angle of depression of 60°. If the height of the lighthouse is 90 metre, then find how far the ship is from the lighthouse.
Chapter: [0.06] Trigonometry
Find the height of an equilateral triangle having side 2a.
Chapter: [0.02] Pythagoras Theorem
Advertisements
In ∆PQR, PM = 15, PQ = 25 PR = 20, NR = 8. State whether line NM is parallel to side RQ. Give reason.

Chapter: [0.01] Similarity
In the adjoining figure, O is the centre of the circle. From point R, seg RM and seg RN are tangent segments touching the circle at M and N. If (OR) = 10 cm and radius of the circle = 5 cm, then
- What is the length of each tangent segment?
- What is the measure of ∠MRO?
- What is the measure of ∠MRN?

Chapter: [0.03] Circle
Draw a circle with radius 3.4 cm. Draw a chord MN of length 5.7 cm in it. construct tangents at point M and N to the circle.
Chapter: [0.04] Geometric Constructions
Find the co-ordinates of the points of trisection of the line segment AB with A(2, 7) and B(–4, –8).
Chapter: [0.04] Geometric Constructions [0.05] Co-ordinate Geometry
In the given figure, the circles with centres P and Q touch each other at R. A line passing through R meets the circles at A and B respectively. Prove that – (1) seg AP || seg BQ,
(2) ∆APR ~ ∆RQB, and
(3) Find ∠ RQB if ∠ PAR = 35°
Chapter: [0.03] Circle
In the given figure shows a toy. Its lower part is a hemisphere and the upper part is a cone. Find the volume and surface area of the toy from the measures shown in the figure (\[\pi = 3 . 14\])
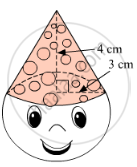
Chapter: [0.07] Mensuration
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 8x − 7, DB = 5x − 3, AE = 4x − 3 and EC = (3x − 1), find the value of x.
Chapter: [0.01] Similarity
Prove that the tangents drawn at the ends of a diameter of a circle are parallel.
Chapter: [0.03] Circle
A solid is in the form of a right circular cylinder, with a hemisphere at one end and a cone at the other end. The radius of the common base is 3.5 cm and the heights of the cylindrical and conical portions are 10 cm. and 6 cm, respectively. Find the total surface area of the solid. (Use π =`22/7`)
Chapter:
Draw a circle of radius 3.4 cm and centre E. Take a point F on the circle. Take another point A such that E-F-A and FA = 4.1 cm. Draw tangents to the circle from point A.
Chapter: [0.04] Geometric Constructions
If A(–14, –10), B(6, –2) is given, find the coordinates of the points which divide segment AB into four equal parts.
Chapter: [0.04] Geometric Constructions [0.05] Co-ordinate Geometry
Other Solutions
Submit Question Paper
Help us maintain new question papers on Shaalaa.com, so we can continue to help studentsonly jpg, png and pdf files
Maharashtra State Board previous year question papers 10th Standard Board Exam Geometry Mathematics 2 with solutions 2019 - 2020
Previous year Question paper for Maharashtra State Board 10th Standard Board Exam Geometry Maths 2-2020 is solved by experts. Solved question papers gives you the chance to check yourself after your mock test.
By referring the question paper Solutions for Geometry Mathematics 2, you can scale your preparation level and work on your weak areas. It will also help the candidates in developing the time-management skills. Practice makes perfect, and there is no better way to practice than to attempt previous year question paper solutions of Maharashtra State Board 10th Standard Board Exam.
How Maharashtra State Board 10th Standard Board Exam Question Paper solutions Help Students ?
• Question paper solutions for Geometry Mathematics 2 will helps students to prepare for exam.
• Question paper with answer will boost students confidence in exam time and also give you an idea About the important questions and topics to be prepared for the board exam.
• For finding solution of question papers no need to refer so multiple sources like textbook or guides.
