Advertisements
Advertisements
प्रश्न
A circle touches the side BC of a ΔABC at a point P and touches AB and AC when produced at Q and R respectively. As shown in the figure that AQ = `1/2` (Perimeter of ΔABC).

उत्तर
We have to prove that
AQ = `1/2` (perimeter of ΔABC)
Perimeter of ΔABC = AB + BC + CA
= AB + BP + PC + CA
= AB + BQ + CR + CA
(∵ Length of tangents from an external point to a circle are equal ∴ BP = BQ and PC = CR)
= AQ + AR ...(∵ AB + BQ = AQ and CR + CA = AR)
= AQ + AQ ...(∵ Length of tangents from an external point are equal)
= 2AQ
`\implies` AQ = `1/2` (Perimeter of ΔABC)
Hence proved.
संबंधित प्रश्न
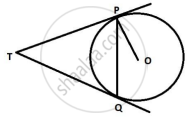
Two tangents TP and TQ are drawn to a circle with centre O from an external point T. Prove that ∠PTQ = 2∠OPQ.
If PT is a tangent at T to a circle whose center is O and OP = 17 cm, OT = 8 cm. Find the length of tangent segment PT.
From an external point P, tangents PA and PB are drawn to the circle with centre O. If CD is the tangent to the circle at point E and PA = 14 cm. Find the perimeter of ABCD.
In following figure, three circles each of radius 3.5 cm are drawn in such a way that each of them touches the other two. Find the area enclosed between these three circles (shaded region). `["Use" pi=22/7]`

A chord of length 14 cm is at a distance of 6 cm from the centre of a circle. The length of another chord at a distance of 2 cm from the centre of the circle is
Use the figure given below to fill in the blank:
AB is a ______ of the circle.

In a circle with centre P, chord AB is parallel to a tangent and intersects the radius drawn from the point of contact to its midpoint. If AB = `16sqrt(3)`, then find the radius of the circle
A point P is 13 cm from the centre of the circle. The length of the tangent drawn from P to the circle is 12cm. Find the radius of the circle.
A point P is 10 cm from the center of a circle. The length of the tangent drawn from P to the circle is 8 cm. The radius of the circle is equal to ______
AB and AC are two equal chords of a circle. Prove that the bisector of the angle BAC passes through the centre of the circle.
