Advertisements
Advertisements
प्रश्न
A ladder is placed against a vertical tower. If the ladder makes an angle of 30° with the ground and reaches upto a height of 18 m of the tower; find length of the ladder.
उत्तर
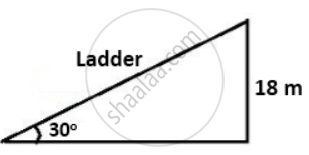
Suppose the length of ladder is x m.
From the figure, we have
`(18)/x` = sin30° ....`[∵ sin = "Perpendicular"/"Hypotenuse"]`
⇒ `(18)/x = (1)/(2)`
⇒ x = 36
Thus, the length of ladder is 36m.
APPEARS IN
संबंधित प्रश्न
If sin 3A = 1 and 0 < A < 90°, find cos 2A
If sin 3A = 1 and 0 < A < 90°, find `tan^2A - (1)/(cos^2 "A")`
Solve for x : cos (2x - 30°) = 0
Solve for 'θ': `sec(θ/2 + 10°) = (2)/sqrt(3)`
In a trapezium ABCD, as shown, AB ‖ DC, AD = DC = BC = 24 cm and ∠A = 30°. Find: length of AB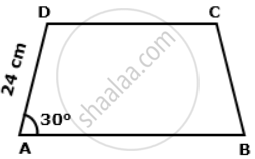
Find:
a. BC
b. AD
c. AC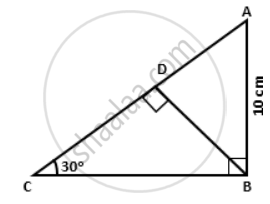
Find the value of 'y' if `sqrt(3)` = 1.723.
Given your answer correct to 2 decimal places.
Evaluate the following: `(sec34°)/("cosec"56°)`
Evaluate the following: sin28° sec62° + tan49° tan41°
Prove the following: tanθ tan(90° - θ) = cotθ cot(90° - θ)
