Advertisements
Advertisements
प्रश्न
Evaluate the following: sin28° sec62° + tan49° tan41°
उत्तर
sin28° sec62° + tan49° tan41°
= sin28° sec(90° - 28°) + tan49° tan(90° - 49°)
= sin28° cosec28° + tan49° cot49°
= `sin28° xx (1)/(sin28°) + tan49° xx (1)/(tan49°)`
= 1 + 1
= 2.
APPEARS IN
संबंधित प्रश्न
If 4 cos2 x = 3 and x is an acute angle;
find the value of :
(i) x
(ii) cos2 x + cot2 x
(iii) cos 3x (iv) sin 2x
If sin 3A = 1 and 0 < A < 90°, find `tan^2A - (1)/(cos^2 "A")`
Solve for x : sin (x + 10°) = `(1)/(2)`
Solve for x : sin2 x + sin2 30° = 1
Find the value of 'A', if (2 - cosec 2A) cos 3A = 0
If A = B = 60°, verify that: cos(A - B) = cosA cosB + sinA sinB
If `sqrt(3)`sec 2θ = 2 and θ< 90°, find the value of θ
Find the value of 'x' in each of the following: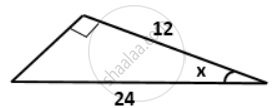
In the given figure, a rocket is fired vertically upwards from its launching pad P. It first rises 20 km vertically upwards and then 20 km at 60° to the vertical. PQ represents the first stage of the journey and QR the second. S is a point vertically below R on the horizontal level as P, find:
a. the height of the rocket when it is at point R.
b. the horizontal distance of point S from P.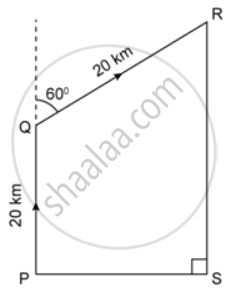
If A, B and C are interior angles of ΔABC, prove that sin`(("A" + "B")/2) = cos "C"/(2)`
