Advertisements
Advertisements
प्रश्न
A uniform cube of mass m and side a is placed on a frictionless horizontal surface. A vertical force F is applied to the edge as shown in figure. Match the following (most appropriate choice):
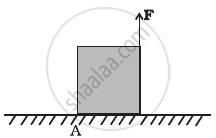
| (a) mg/4 < F < mg/2 | (i) Cube will move up. |
| (b) F > mg/2 | (ii) Cube will not exhibit motion. |
| (c) F > mg | (iii) Cube will begin to rotate and slip at A. |
| (d) F = mg/4 | (iv) Normal reaction effectively at a/3 from A, no motion. |
उत्तर
| (a) mg/4 < F < mg/2 | (ii) Cube will not exhibit motion. |
| (b) F > mg/2 | (iii) Cube will begin to rotate and slip at A. |
| (c) F > mg | (i) Cube will move up. |
| (d) F = mg/4 | (iv) Normal reaction effectively at a/3 from A, no motion. |
Explanation:
Consider the below diagram
Moment of the force F about point A, τ1 = F × a .....(anti-clockwise)
Moment of weight mg of the cube about point A.
τ2 = `mg xx a/2` .....(clockwise)
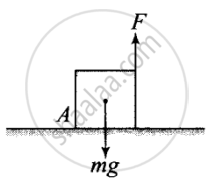
Cube will not exhibit motion, If τ1 = τ2 ......(∵ In this case, both the torque will cancel the effect of each other)
∴ F × a = `mg xx a/2`
⇒ F = `(mg)/2`
Cube will rotate only when, τ1 > τ2
⇒ F × a > `mg xx a/2`
⇒ `F > (mg)/2`
Let the normal reaction is acting at `a/3` from point A, then
`mg xx a/3 = F xx a` or `F = (mg)/3` .......(For no motion)
When F = `(mg)/4` which is less than `(mg)/3`, .....`(F < (mg)/3)`
There will be no motion.
APPEARS IN
संबंधित प्रश्न
The torque of a force \[\overrightarrow F \] about a point is defined as \[\overrightarrow\Gamma = \overrightarrow r \times \overrightarrow F.\] Suppose \[\overrightarrow r, \overrightarrow F\] and \[\overrightarrow \Gamma\] are all nonzero. Is \[r \times \overrightarrow\Gamma || \overrightarrow F\] always true? Is it ever true?
If several forces act on a particle, the total torque on the particle may be obtained by first finding the resultant force and then taking torque of this resultant. Prove this. Is this result valid for the forces acting on different particles of a body in such a way that their lines of action intersect at a common point?
A cubical block of mass m and edge a slides down a rough inclined plane of inclination θ with a uniform speed. Find the torque of the normal force acting on the block about its centre.
A 6⋅5 m long ladder rests against a vertical wall reaching a height of 6⋅0 m. A 60 kg man stands half way up the ladder.
- Find the torque of the force exerted by the man on the ladder about the upper end of the ladder.
- Assuming the weight of the ladder to be negligible as compared to the man and assuming the wall to be smooth, find the force exerted by the ground on the ladder.
A rope is wound around a hollow cylinder of mass 3 kg and radius 40 cm. What is the angular acceleration of the cylinder if the rope is pulled with a force of 30 N?
Define torque and mention its unit.
State conservation of angular momentum.
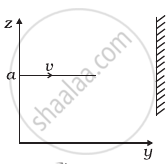
A particle of mass m is moving in yz-plane with a uniform velocity v with its trajectory running parallel to + ve y-axis and intersecting z-axis at z = a (Figure). The change in its angular momentum about the origin as it bounces elastically from a wall at y = constant is ______.
A uniform sphere of mass m and radius R is placed on a rough horizontal surface (Figure). The sphere is struck horizontally at a height h from the floor. Match the following:
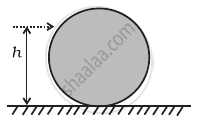
| Column I | Column II | |
| (a) h = R/2 | (i) | Sphere rolls without slipping with a constant velocity and no loss of energy. |
| (b) h = R | (ii) | Sphere spins clockwise, loses energy by friction. |
| (c) h = 3R/2 | (iii) | Sphere spins anti-clockwise, loses energy by friction. |
| (d) h = 7R/5 | (iv) | Sphere has only a translational motion, looses energy by friction. |
A particle of mass 'm' is moving in time 't' on a trajectory given by
`vecr = 10alphat^2hati + 5beta(t - 5)hatj`
Where α and β are dimensional constants.
The angular momentum of the particle becomes the same as it was for t = 0 at time t = ______ seconds.
