Question

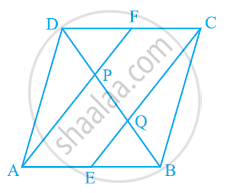
D, E, and F are the mid-points of the sides AB, BC, and CA respectively of ΔABC. AE meets DF at O. P and Q are the mid-points of OB and OC respectively. Prove that DPQF is a parallelogram.

Answer 1

Given: △ABC, D, E, F are midpoints of AB, BC, AC respectively. AB and DF meet at O. P and Q are midpoints of OB and OC respectively.
To Prove: DPFQ is a parallelogram.

Proof:
In △ABC,
D is the mid-point of AB and F is the mid-point of AC
Hence, DF ∥ BC and DF = `1/2​`BC        ... (1) (Mid-point theorem)
In △OBC,
P is the mid-point of OB and Q is the mid-point of OC
Hence, PQ ∥ BC and PQ = `1/2`​BC       ... (2) (mid-point theorem)
thus, from (1) and (2)
DF ∥ PQ and DF = PQ                      ....(3)

Now, In △AOB,
D is the mid-point of AB and P is the mid-point of OB
Thus, DP ∥ AE and DP = `1/2`​AE        ....(4) (midpoint theorem)
 Now, In △AOC,
F is the midpoint of AC and Q is the midpoint of OC
Thus, FQ ∥ AE and QF = `1/2`​AE         .....(5) (midpoint theorem)
thus, from (4) and (5)
DP ∥ FQ and DP = FQ                      .....(6)

DPFQ is a parallelogram                 ......(from (3) and (6))
Hence proved.