Advertisements
Advertisements
प्रश्न
Evaluate `cos[cos^-1 ((-sqrt(3))/2) + pi/6]`
उत्तर
`cos[cos^-1 ((-sqrt(3))/2) + pi/6] = cos[cos^-1(cos (5pi)/6) + pi/6]` ......`(because cos (5pi)/6 = (-sqrt(3))/2)`
= `cos((5pi)/6 + pi/6)` ......`(because cos^-1 cosx = x; x ∈ [0, pi])`
= `cos(pi)` = –1
APPEARS IN
संबंधित प्रश्न
Solve for x : tan-1 (x - 1) + tan-1x + tan-1 (x + 1) = tan-1 3x
If a line makes angles 90°, 60° and θ with x, y and z-axis respectively, where θ is acute, then find θ.
Write the function in the simplest form: `tan^(-1) ((cos x - sin x)/(cos x + sin x)) `,` 0 < x < pi`
Find the value of the given expression.
`tan^(-1) (tan (3pi)/4)`
Prove that:
`sin^(-1) 8/17 + sin^(-1) 3/5 = tan^(-1) 77/36`
sin–1 (1 – x) – 2 sin–1 x = `pi/2` , then x is equal to ______.
If y = `(x sin^-1 x)/sqrt(1 -x^2)`, prove that: `(1 - x^2)dy/dx = x + y/x`
Find the value of the expression in terms of x, with the help of a reference triangle
sin (cos–1(1 – x))
Find the value of `sin^-1[cos(sin^-1 (sqrt(3)/2))]`
Find the value of `tan(sin^-1 3/5 + cot^-1 3/2)`
Evaluate `tan^-1(sin((-pi)/2))`.
Evaluate `cos[sin^-1 1/4 + sec^-1 4/3]`
Show that `2tan^-1 {tan alpha/2 * tan(pi/4 - beta/2)} = tan^-1 (sin alpha cos beta)/(cosalpha + sinbeta)`
Prove that `tan^-1 ((sqrt(1 + x^2) + sqrt(1 - x^2))/((1 + x^2) - sqrt(1 - x^2))) = pi/2 + 1/2 cos^-1x^2`
Show that `tan(1/2 sin^-1 3/4) = (4 - sqrt(7))/3` and justify why the other value `(4 + sqrt(7))/3` is ignored?
`"cot" (pi/4 - 2 "cot"^-1 3) =` ____________.
`"sin" {2 "cos"^-1 ((-3)/5)}` is equal to ____________.
The value of the expression tan `(1/2 "cos"^-1 2/sqrt3)`
`"tan" (pi/4 + 1/2 "cos"^-1 "x") + "tan" (pi/4 - 1/2 "cos"^-1 "x") =` ____________.
`"cos"^-1["cos"(2"cot"^-1(sqrt2 - 1))]` = ____________.
`"sin"^-1 (1/sqrt2)`
`"cos"^-1 (1/2)`
The Government of India is planning to fix a hoarding board at the face of a building on the road of a busy market for awareness on COVID-19 protocol. Ram, Robert and Rahim are the three engineers who are working on this project. “A” is considered to be a person viewing the hoarding board 20 metres away from the building, standing at the edge of a pathway nearby. Ram, Robert and Rahim suggested to the firm to place the hoarding board at three different locations namely C, D and E. “C” is at the height of 10 metres from the ground level. For viewer A, the angle of elevation of “D” is double the angle of elevation of “C” The angle of elevation of “E” is triple the angle of elevation of “C” for the same viewer. Look at the figure given and based on the above information answer the following:
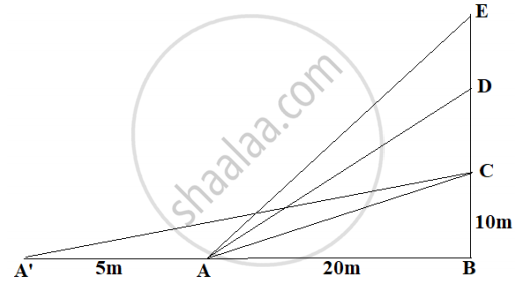
Measure of ∠EAB = ________.
The Government of India is planning to fix a hoarding board at the face of a building on the road of a busy market for awareness on COVID-19 protocol. Ram, Robert and Rahim are the three engineers who are working on this project. “A” is considered to be a person viewing the hoarding board 20 metres away from the building, standing at the edge of a pathway nearby. Ram, Robert and Rahim suggested to the firm to place the hoarding board at three different locations namely C, D and E. “C” is at the height of 10 metres from the ground level. For viewer A, the angle of elevation of “D” is double the angle of elevation of “C” The angle of elevation of “E” is triple the angle of elevation of “C” for the same viewer. Look at the figure given and based on the above information answer the following:

𝐴' Is another viewer standing on the same line of observation across the road. If the width of the road is 5 meters, then the difference between ∠CAB and ∠CA'B is ______.
The Government of India is planning to fix a hoarding board at the face of a building on the road of a busy market for awareness on COVID-19 protocol. Ram, Robert and Rahim are the three engineers who are working on this project. “A” is considered to be a person viewing the hoarding board 20 metres away from the building, standing at the edge of a pathway nearby. Ram, Robert and Rahim suggested to the firm to place the hoarding board at three different locations namely C, D and E. “C” is at the height of 10 metres from the ground level. For viewer A, the angle of elevation of “D” is double the angle of elevation of “C” The angle of elevation of “E” is triple the angle of elevation of “C” for the same viewer. Look at the figure given and based on the above information answer the following:

Domain and Range of tan-1 x = ________.
`tan(2tan^-1 1/5 + sec^-1 sqrt(5)/2 + 2tan^-1 1/8)` is equal to ______.
If sin–1x + sin–1y + sin–1z = π, show that `x^2 - y^2 - z^2 + 2yzsqrt(1 - x^2) = 0`
Solve:
sin–1(x) + sin–1(1 – x) = cos–1x.
