Advertisements
Advertisements
प्रश्न
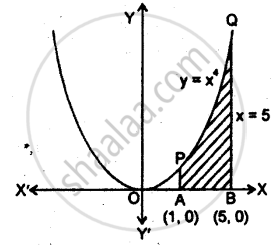
Find the area under the given curve and given line:
y = x4, x = 1, x = 5 and x-axis
उत्तर
The curve y = x4 passes through the point (0, 0). The line OY is symmetric.
Now, y = x4
`dy/dx = 4x^3`
The sign of `dy/dx` changes from -ve to +ve when x moves through x = 0.
∴ x = 0 is the lowest point.
∴ Area of the region bounded by y = x4, x = 1, x = 5 and x-axis
= Area of the region PABQ
`= int_1^5 y dx = int_1^5 x^4 dx`
`= [x^5/5]_1^5 = [5^5/5 - 1/5]`
`= [5^4 - 1/5]`
`= 625 - 1/5`
`= (3125 - 1)/5`
`= 3124/5`
= 624.8 square unit
APPEARS IN
संबंधित प्रश्न
Find the area of the region bounded by x2 = 4y, y = 2, y = 4 and the y-axis in the first quadrant.
Find the area of the region in the first quadrant enclosed by x-axis, line x = `sqrt3` y and the circle x2 + y2 = 4.
Find the area of the region bounded by the curve y2 = 4x and the line x = 3
Find the area under the given curve and given line:
y = x2, x = 1, x = 2 and x-axis
Find the area between the curves y = x and y = x2
Sketch the graph of y = |x + 3| and evaluate `int_(-6)^0 |x + 3|dx`
Find the area enclosed by the parabola 4y = 3x2 and the line 2y = 3x + 12
Find the area of the smaller region bounded by the ellipse `x^2/a^2 + y^2/b^2 = 1` and the line `x/a + y/b = 1`
Find the area of the region enclosed by the parabola x2 = y, the line y = x + 2 and x-axis
Using the method of integration find the area of the triangle ABC, coordinates of whose vertices are A(2, 0), B (4, 5) and C (6, 3).
Using the method of integration, find the area of the triangle ABC, coordinates of whose vertices are A (4 , 1), B (6, 6) and C (8, 4).
Find the area enclosed between the parabola 4y = 3x2 and the straight line 3x - 2y + 12 = 0.
Find the area of the smaller region bounded by the ellipse \[\frac{x^2}{9} + \frac{y^2}{4} = 1\] and the line \[\frac{x}{3} + \frac{y}{2} = 1 .\]
Draw a rough sketch and find the area bounded by the curve x2 = y and x + y = 2.
Find the area of the region bounded by the following curves, the X-axis and the given lines: y = x4, x = 1, x = 5
Find the area of the region bounded by the following curves, the X-axis and the given lines: 2y = 5x + 7, x = 2, x = 8
Find the area of the region bounded by the following curves, the X-axis and the given lines: 2y + x = 8, x = 2, x = 4
Find the area of the region bounded by the following curves, the X-axis and the given lines:
y = x2 + 1, x = 0, x = 3
Choose the correct alternative :
Area of the region bounded by the curve x2 = y, the X-axis and the lines x = 1 and x = 3 is _______.
State whether the following is True or False :
The area bounded by the curve x = g (y), Y-axis and bounded between the lines y = c and y = d is given by `int_"c"^"d"x*dy = int_(y = "c")^(y = "d") "g"(y)*dy`
State whether the following is True or False :
The area bounded by the two cures y = f(x), y = g (x) and X-axis is `|int_"a"^"b" f(x)*dx - int_"b"^"a" "g"(x)*dx|`.
Solve the following:
Find the area of the region bounded by the curve x2 = 25y, y = 1, y = 4 and the Y-axis.
The area of the shaded region bounded by two curves y = f(x), and y = g(x) and X-axis is `int_"a"^"b" "f"(x) "d"x + int_"a"^"b" "g"(x) "d"x`
The area of the region bounded by the curve y2 = 4x, the X axis and the lines x = 1 and x = 4 is ______
The area of the region lying in the first quadrant and bounded by the curve y = 4x2, and the lines y = 2 and y = 4 is ______
The area of the region bounded by y2 = 25x, x = 1 and x = 2 the X axis is ______
Find area of the region bounded by 2x + 4y = 10, y = 2 and y = 4 and the Y-axis lying in the first quadrant
Find area of the region bounded by the curve y = – 4x, the X-axis and the lines x = – 1 and x = 2
Area bounded by the curve xy = 4, X-axis between x = 1, x = 5 is ______.
Area under the curve `y=sqrt(4x+1)` between x = 0 and x = 2 is ______.
Which equation below represents a parabola that opens upward with a vertex at (0, – 5)?
The area of the circle `x^2 + y^2 = 16`, exterior to the parabola `y = 6x`
The area (in sq.units) of the part of the circle x2 + y2 = 36, which is outside the parabola y2 = 9x, is ______.
Find the area of the region lying in the first quadrant and bounded by y = 4x2, x = 0,y = 2 and y = 4.
Find the area of the regions bounded by the line y = −2x, the X-axis and the lines x = −1 and x = 2.
