Advertisements
Advertisements
प्रश्न
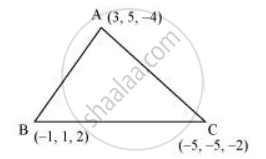
Find the Direction Cosines of the Sides of the triangle Whose Vertices Are (3, 5, -4), (-1, 1, 2) and (-5, -5, -2).
उत्तर
Let A(3, 5,−4), B(−1, 1, 2) and C(−5, −5, −2).
Direction ratio of AB = (−1 − 3), (1 − 5), (2 − (−4))
= (−4, −4, 6)
|AB| = `sqrt((-4)^2 + (-4)^2 + (6)^2)`
= `sqrt(16 + 16 + 36)`
= `sqrt68`
= `2sqrt17`
Direction ratio of BC = (−5 − (−1), −5 − 1, −2 − 2)
= (−4, −6, −4)
|BC| = `sqrt((-4)^2 + (-6)^2 + (-4)^2)`
= `sqrt(16 + 36 + 16)`
= `sqrt68`
= `2sqrt17`
Direction ratio of CA = (−5 − 3, −5 − 5, −2 − (−4))
= (−8, −10, 2)
|CA| = `sqrt((-8)^2 + (-10)^2 + (2)^2)`
= `sqrt(64 + 100 + 4)`
= `sqrt168`
= `2sqrt42`
∴ AB are `< (-1 - 3)/|AB|, (1 - 5)/|AB|, (2 + 4)/|AB| >`
i.e., `< (-2)/sqrt17, (-2)/sqrt17, 3/sqrt17 >`
∴ d.c. of BC are `< (-5 + 1)/|BC|, (- 5 - 1)/|BC|, (- 2 -2)/|BC|>`
i.e., `< (-2)/sqrt17, (-3)/sqrt17, (-2)/sqrt17 >`
∴ d.c of CA are `< (3 + 5)/|CA|, (5 + 5)/|CA|, (- 4 + 2)/|CA|`
i.e., `< 4/sqrt42, 5/sqrt42, (-1)/sqrt42 >`
APPEARS IN
संबंधित प्रश्न
Find the direction cosines of the line perpendicular to the lines whose direction ratios are -2, 1,-1 and -3, - 4, 1
Which of the following represents direction cosines of the line :
(a)`0,1/sqrt2,1/2`
(b)`0,-sqrt3/2,1/sqrt2`
(c)`0,sqrt3/2,1/2`
(d)`1/2,1/2,1/2`
If a line makes angles 90°, 135°, 45° with the X, Y, and Z axes respectively, then its direction cosines are _______.
(A) `0,1/sqrt2,-1/sqrt2`
(B) `0,-1/sqrt2,-1/sqrt2`
(C) `1,1/sqrt2,1/sqrt2`
(D) `0,-1/sqrt2,1/sqrt2`
Find the angle between the lines whose direction ratios are 4, –3, 5 and 3, 4, 5.
Show that the points (2, 3, 4), (−1, −2, 1), (5, 8, 7) are collinear.
If l1, m1, n1 and l2, m2, n2 are the direction cosines of two mutually perpendicular lines, show that the direction cosines of the line perpendicular to both of these are m1n2 − m2n1, n1l2 − n2l1, l1m2 − l2m1.
If the lines `(x-1)/(-3) = (y -2)/(2k) = (z-3)/2 and (x-1)/(3k) = (y-1)/1 = (z -6)/(-5)` are perpendicular, find the value of k.
If a line makes angles of 90°, 60° and 30° with the positive direction of x, y, and z-axis respectively, find its direction cosines
Using direction ratios show that the points A (2, 3, −4), B (1, −2, 3) and C (3, 8, −11) are collinear.
If the coordinates of the points A, B, C, D are (1, 2, 3), (4, 5, 7), (−4, 3, −6) and (2, 9, 2), then find the angle between AB and CD.
Find the angle between the lines whose direction cosines are given by the equations
l + 2m + 3n = 0 and 3lm − 4ln + mn = 0
Define direction cosines of a directed line.
What are the direction cosines of Y-axis?
What are the direction cosines of Z-axis?
Write the angle between the lines whose direction ratios are proportional to 1, −2, 1 and 4, 3, 2.
Write the coordinates of the projection of the point P (2, −3, 5) on Y-axis.
Write direction cosines of a line parallel to z-axis.
For every point P (x, y, z) on the xy-plane,
A parallelopiped is formed by planes drawn through the points (2, 3, 5) and (5, 9, 7), parallel to the coordinate planes. The length of a diagonal of the parallelopiped is
The angle between the two diagonals of a cube is
The direction ratios of the line which is perpendicular to the lines with direction ratios –1, 2, 2 and 0, 2, 1 are _______.
Verify whether the following ratios are direction cosines of some vector or not
`4/3, 0, 3/4`
Find the direction cosines and direction ratios for the following vector
`3hat"i" + hat"j" + hat"k"`
Find the direction cosines and direction ratios for the following vector
`5hat"i" - 3hat"j" - 48hat"k"`
Find the direction cosines and direction ratios for the following vector
`3hat"i" - 3hat"k" + 4hat"j"`
Find the direction cosines and direction ratios for the following vector
`hat"i" - hat"k"`
If (a, a + b, a + b + c) is one set of direction ratios of the line joining (1, 0, 0) and (0, 1, 0), then find a set of values of a, b, c
Choose the correct alternative:
The unit vector parallel to the resultant of the vectors `hat"i" + hat"j" - hat"k"` and `hat"i" - 2hat"j" + hat"k"` is
P is a point on the line segment joining the points (3, 2, –1) and (6, 2, –2). If x co-ordinate of P is 5, then its y co-ordinate is ______.
If a line makes angles α, β, γ with the positive directions of the coordinate axes, then the value of sin2α + sin2β + sin2γ is ______.
If a line makes an angle of `pi/4` with each of y and z-axis, then the angle which it makes with x-axis is ______.
The vector equation of the line passing through the points (3, 5, 4) and (5, 8, 11) is `vec"r" = 3hat"i" + 5hat"j" + 4hat"k" + lambda(2hat"i" + 3hat"j" + 7hat"k")`
If the directions cosines of a line are k,k,k, then ______.
What will be the value of 'P' so that the lines `(1 - x)/3 = (7y - 14)/(2P) = (z - 3)/2` and `(7 - 7x)/(3P) = (y - 5)/1 = (6 - z)/5` at right angles.
The co-ordinates of the point where the line joining the points (2, –3, 1), (3, –4, –5) cuts the plane 2x + y + z = 7 are ______.
If two straight lines whose direction cosines are given by the relations l + m – n = 0, 3l2 + m2 + cnl = 0 are parallel, then the positive value of c is ______.
Equation of line passing through origin and making 30°, 60° and 90° with x, y, z axes respectively, is ______.
If a line makes an angle α, β and γ with positive direction of the coordinate axes, then the value of sin2α + sin2β + sin2γ will be ______.
