Advertisements
Advertisements
प्रश्न
Find the missing values in the following table for the circles with radius (r), diameter (d) and Circumference (C).
| radius (r) | diameter (d) | Circumference (C) |
| 1760 cm |
उत्तर
Given: Circumference C = 1760 cm
2πr = 1760
`2 xx 22/7 xx "r"` = 1760
r = `(1760 xx 7)/(2 xx 22)`
= `(160 xx 7)/(2 xx 2)`
= 40 × 7
= 280 cm
diameter = 2 × r
= 2 × 280
= 560 cm
Tabulating the results
| radius (r) | diameter (d) | Circumference (C) |
| 280 cm | 560 cm | 1760 cm |
APPEARS IN
संबंधित प्रश्न
Find the length of a tangent drawn to a circle with radius 5cm, from a point 13 cm from the center of the circle.
Prove that the intercept of a tangent between two parallel tangents to a circle subtends a right angle at center.
From an external point P, tangents PA and PB are drawn to the circle with centre O. If CD is the tangent to the circle at point E and PA = 14 cm. Find the perimeter of ABCD.
Draw different pairs of circles. How many points does each pair have in common? What is the maximum number of common points?
If O is the centre of a circle of radius r and AB is a chord of the circle at a distance r/2 from O, then ∠BAO =
The length of three concesutive sides of a quadrilateral circumscribing a circle are 4 cm, 5 cm, and 7 cm respectively. Determine the length of the fourth side.
In the given figure, the area enclosed between the two concentric circles is 770 cm2. If the radius of the outer circle is 21 cm, calculate the radius of the inner circle.
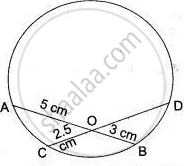
In Fig., chords AB and CD of the circle intersect at O. AO = 5 cm, BO = 3 cm and CO = 2.5 cm. Determine the length of DO.
Use the figure given below to fill in the blank:
Diameter = 2 x ________

The diameter of the circle is 52 cm and the length of one of its chord is 20 cm. Find the distance of the chord from the centre
In a circle, AB and CD are two parallel chords with centre O and radius 10 cm such that AB = 16 cm and CD = 12 cm determine the distance between the two chords?
The longest chord of a circle is __________
The radius of a circle of diameter 24 cm is _______
Given: A circle inscribed in a right angled ΔABC. If ∠ACB = 90° and the radius of the circle is r.
To prove: 2r = a + b – c
If the angle between two tangents drawn from a point P to a circle of radius ‘a’ and centre ‘O’ is 90°, then OP = ______
A point P is 13 cm from the centre of the circle. The length of the tangent drawn from P to the circle is 12cm. Find the radius of the circle.
If a hexagon ABCDEF circumscribe a circle, prove that AB + CD + EF = BC + DE + FA.
In the following figure, ∠ADC = 130° and chord BC = chord BE. Find ∠CBE.

From the figure, identify the centre of the circle.

From the figure, identify three radii.