Advertisements
Advertisements
प्रश्न
If 3 cos A = 4 sin A, find the value of :
(i) cos A(ii) 3 - cot2 A + cosec2A.
उत्तर
Consider the diagram below :
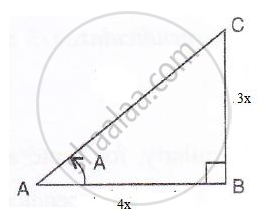
3cos A = 4 sin A
cot A = `(4)/(3)`
i.e. `"base"/"perpendicular" = (4)/(3) ⇒ "AB"/"BC" = (4)/(3)`
Therefore if length of AB = 4x, length of BC = 3x
Since
AB2 + BC = AC2 ...[ Using Pythagoras Theorem]
(4x)2 + (3x)2 = AC2
AC2 = 25x2
∴ AC = 5x ...( hypotenuse)
(i) cos A = `"AB"/"AC" = (4)/(5)`
(ii) cosec A = `"AC"/"BC" = (5)/(3)`
Therefore
3–cot2 A + cosec2 A
= `3 – (4/3)^2+(5/3)^2`
= `(27 – 16 + 25)/(9)`
=`(36)/(9)`
= 4
APPEARS IN
संबंधित प्रश्न
In rectangle ABCD AB = 20cm ∠BAC = 60° BC, calculate side BC and diagonals AC and BD.
If 3tan θ 4 , show that `((4cos theta - sin theta ))/((4 cos theta + sin theta))=4/5`
If sin (A – B) = `1/2` and cos (A + B) = `1/2, 0^0 ≤ (A + B) ≤ 90^0` and A > B, then find A and B.
sin20° = cos ______°
In a right-angled triangle, it is given that A is an acute angle and tan A = `(5) /(12)`.
find the value of :
(i) cos A
(ii) sin A
(iii) ` (cosA+sinA)/(cosA– sin A)`
In an isosceles triangle ABC, AB = BC = 6 cm and ∠B = 90°. Find the values of cosec C
If sin A = `(7)/(25)`, find the value of : `"cos A" + (1)/"cot A"`
If sin θ = `"a"/sqrt("a"^2 + "b"^2)`, then show that b sin θ = a cos θ
If cos θ : sin θ = 1 : 2, then find the value of `(8costheta - 2sintheta)/(4costheta + 2sintheta`
Statement A (Assertion): For 0 < θ ≤ 90°, cosec θ – cot θ and cosec θ + cot θ are reciprocal of each other.
Statement R (Reason): cosec2 θ – cot2 θ = 1
