Advertisements
Advertisements
प्रश्न
If tan (A + B) = 1 and tan(A-B)`=1/sqrt3` , 0° < A + B < 90°, A > B, then find the values of A and B.
उत्तर
tan (A+B) = 1
⇒ tan(A+B) = tan 45°
⇒ A +B = 45° ..........(1)
tan(A-B)`=1/sqrt3`
⇒ tan (A-B)=tan 30°
⇒ A - B = 30 ......(2)
On adding (1) and (2), we obtain
2A = 75°
⇒ A = 37.5°
Putting the value of A in (1) we get
37.5° + B = 45°
⇒ B = 7.5°
Therefore,∠A = 37.5° and ∠B = 7.5°
APPEARS IN
संबंधित प्रश्न
Evaluate the following:
`(cos 45°)/(sec 30° + cosec 30°)`
Evaluate the following
`sec 11^@/(cosec 79^@)`
Evaluate the following :
`((sin 27^@)/(cos 63^@))^2 - (cos 63^@/sin 27^@)^2`
Evaluate: `(3 cos 55^@)/(7 sin 35^@) - (4(cos 70 cosec 20^@))/(7(tan 5^@ tan 25^@ tan 45^@ tan 65^@ tan 85^@))`
Evaluate:
`(cos3"A" – 2cos4"A")/(sin3"A" + 2sin4"A")` , when A = 15°
ABC is an isosceles right-angled triangle. Assuming of AB = BC = x, find the value of each of the following trigonometric ratios: tan 45°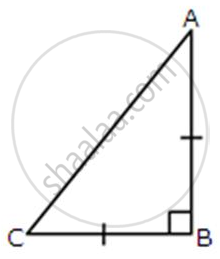
If sec A = cosec A and 0° ∠A ∠90°, state the value of A
If A =30o, then prove that :
cos 2A = cos2A - sin2A = `(1 – tan^2"A")/(1+ tan^2"A")`
If 2 sin 2θ = `sqrt(3)` then the value of θ is
If sin α = `1/2`, then find the value of (3 cos α – 4 cos3 α).
