Advertisements
Advertisements
प्रश्न
In a cyclic-quadrilateral PQRS, angle PQR = 135°. Sides SP and RQ produced meet at point A whereas sides PQ and SR produced meet at point B. If ∠A : ∠B = 2 : 1; find angles A and B.
उत्तर
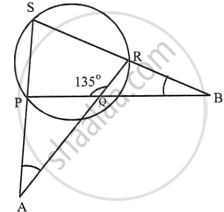
PQRS is a cyclic quadrilateral in which ∠PQR = 135°
Sides SP and RQ are produced to meet at A and sides PQ and SR are produced to meet at B.
∠A : ∠B = 2 : 1
Let ∠A = 2x, then ∠B = x
Now, in cyclic quad PQRS,
Since, ∠PQR = 135°, ∠S = 180° – 135° = 45°
[Since sum of opposite angles of a cyclic quadrilateral are supplementary]
Since, ∠PQR and ∠PQA are linear pair,
∠PQR + ∠PQA = 180°
`=>` 135° + ∠PQA = 180°
`=>` ∠PQA = 180° – 135° = 45°
Now, In ∆PBS,
∠P = 180° – (45° + x)
= 180° – 45° – x
= 135° – x ...(1)
Again, in ∆PQA,
EXT ∠P = ∠PQA + ∠A
= 45° + 2x ...(2)
From (1) and (2),
45° + 2x = 135° – x
`=>` 2x + x = 135° – 45°
`=>` 3x = 90°
`=>` x = 30°
Hence, ∠A = 2x = 2 × 30° = 60° and ∠B = x = 30°
APPEARS IN
संबंधित प्रश्न
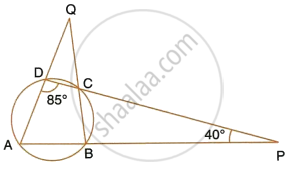
In the figure, ABCD is a cyclic quadrilateral with BC = CD. TC is tangent to the circle at point C and DC is produced to point G. If ∠BCG = 108° and O is the centre of the circle, find :
- angle BCT
- angle DOC

Use the given figure to find:
- ∠BAD,
- ∠DQB.
In the following figure, ABCD is a cyclic quadrilateral in which AD is parallel to BC.

If the bisector of angle A meets BC at point E and the given circle at point F, prove that:
- EF = FC
- BF = DF
In a cyclic quadrialteral ABCD , if m ∠ A = 3 (m ∠C). Find m ∠ A.
In a circle with centre O , chords AB and CD intersets inside the circle at E . Prove that ∠ AOC = ∠ BOD = 2 ∠ AEC.
The bisectors of the opposite angles A and C of a cydic quadrilateral ABCD intersect the cirde at the points E and F, respectively. Prove that EF is a diameter of the circle.
In the given figure, AB is the diameter. The tangent at C meets AB produced at Q.

If ∠ CAB = 34° , find : ∠ CQB
In the figure, given below, find: ∠ADC, Show steps of your working.
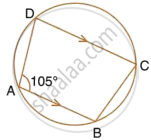
In the figure, given below, find: ∠ABC. Show steps of your working.

In ABCD is a cyclic quadrilateral; O is the centre of the circle. If BOD = 160°, find the measure of BPD.
