Advertisements
Advertisements
प्रश्न
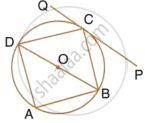
In the given figure, ABCD is a cyclic quadrilateral, PQ is tangent to the circle at point C and BD is its diameter. If ∠DCQ = 40° and ∠ABD = 60°, find;
- ∠DBC
- ∠BCP
- ∠ADB
उत्तर
i. PQ is tangent and CD is a chord
∴ ∠DCQ = ∠DBC ...(Angles in the alternate segment)
∴ DBC = 40° ...(∵ ∠DCQ = 40°)
ii. ∠DCQ + ∠DCB + ∠BCP = 180°
`=>` 40° + 90° + ∠BCP = 180° ...(∵ ∠DCB = 90°)
`=>` 130° + ∠BCP = 180°
∴ ∠BCP = 180° – 130° = 50°
iii. In ΔABD,
∠BAD = 90°, ∠ABD = 60°
∴ ∠ADB = 180° – (90° + 60°)
`=>` ∠ADB = 180° – 150° = 30°
APPEARS IN
संबंधित प्रश्न
In the figure, given below, find:
- ∠BCD,
- ∠ADC,
- ∠ABC.
Show steps of your working.

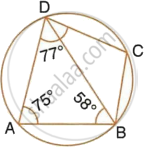
In the figure, given below, ABCD is a cyclic quadrilateral in which ∠BAD = 75°; ∠ABD = 58° and ∠ADC = 77°. Find:
- ∠BDC,
- ∠BCD,
- ∠BCA.
ABCDE is a cyclic pentagon with centre of its circumcircle at point O such that AB = BC = CD and angle ABC = 120°.
Calculate:
- ∠BEC
- ∠BED
D and E are points on equal sides AB and AC of an isosceles triangle ABC such that AD = AE. Prove that the points B, C, E and D are concyclic.
In fig., O is the centre of the circle and ∠ AOC = 1500. Find ∠ ABC.
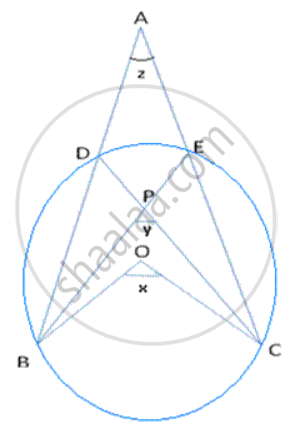
In following fig., O is the centre of the circle, prove that ∠x =∠ y + ∠ z.
In following figure , Δ PQR is an isosceles teiangle with PQ = PR and m ∠ PQR = 35° .Find m ∠ QSR and ∠ QTR

In the figure, given below, find: ∠ABC. Show steps of your working.

In cyclic quadrilateral ABCD, ∠DAC = 27°; ∠DBA = 50° and ∠ADB = 33°. Calculate : ∠CAB.

In the figure , Δ PQR is an isosceles triangle with PQ = PR, and m ∠ PQR = 35°. Find m ∠ QSR and ∠ QTR.
