Advertisements
Advertisements
प्रश्न
In the given figure, O is the centre of the circle. m ( arc PQR) = 60° OP = 10 cm. Find the area of the shaded region.( \[\pi\]= 3.14, \[\sqrt{3}\]= 1.73)

उत्तर
Radius of the circle, r = 10 cm
m(arc PQR) = ∠POR = θ = 60º
∴ Area of the shaded region = Area of segment PQR
\[= r^2 \left( \frac{\pi\theta}{360°} - \frac{\sin\theta}{2} \right)\]
\[ = \left( 10 \right)^2 \left( \frac{3 . 14 \times 60°}{360°} - \frac{\sin60°}{2} \right)\]
\[ = 100 \times \left( \frac{3 . 14}{6} - \frac{\sqrt{3}}{4} \right)\]
\[ = \frac{314}{6} - \frac{173}{4} \left( \sqrt{3} = 1 . 73 \right)\]
\[ = 52 . 33 - 43 . 25\]
\[ = 9 . 08 {cm}^2\]
Thus, the area of the shaded region is 9.08 cm2.
APPEARS IN
संबंधित प्रश्न
A chord of a circle of radius 12 cm subtends an angle of 120° at the centre. Find the area of the corresponding segment of the circle. [Use π = 3.14 and `sqrt3 = 1.73` ]
To warn ships for underwater rocks, a lighthouse spreads a red coloured light over a sector of angle 80° to a distance of 16.5 km. Find the area of the sea over which the ships warned. [Use π = 3.14]
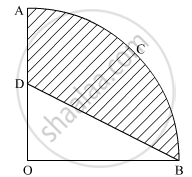
In the given figure, OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD = 2 cm, find the area of the shaded region.
A sector of circle of radius 4cm contains an angle of 30°. Find the area of sector
The diagram shows a sector of circle of radius ‘r’ can containing an angle 𝜃. The area of sector is A cm2 and perimeter of sector is 50 cm. Prove that
(i) 𝜃 =`360/pi(25/r− 1)`
(ii) A = 25r – r2

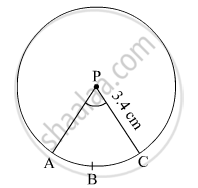
In the given figure, radius of circle is 3.4 cm and perimeter of sector P-ABC is 12.8 cm . Find A(P-ABC).
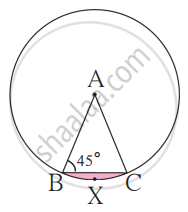
In the given figure, A is the center of the circle. ∠ABC = 45° and AC = 7√2 cm. Find the area of segment BXC.
In the given figure, if A is the centre of the circle. \[\angle\] PAR = 30°, AP = 7.5, find the area of the segment PQR. (\[\pi\] = 3.14)

If angle of sector is 60°, radius is 3.5 cm then length of the arc is ____________.
Find the area of the shaded region where ABC is a quadrant of radius 5cm and a semicircle is drawn with BC as diameter.

The area of the shaded portion in the following figure is equal to the area of.
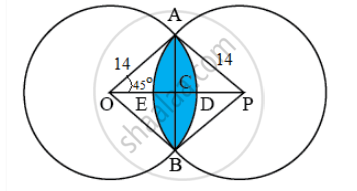
In figure, a square is inscribed in a circle of diameter d and another square is circumscribing the circle. Is the area of the outer square four times the area of the inner square? Give reasons for your answer.
The areas of two sectors of two different circles with equal corresponding arc lengths are equal. Is this statement true? Why?
Find the area of the minor segment of a circle of radius 14 cm, when the angle of the corresponding sector is 60°.
In figure, arcs are drawn by taking vertices A, B and C of an equilateral triangle of side 10 cm. to intersect the sides BC, CA and AB at their respective mid-points D, E and F. Find the area of the shaded region (Use π = 3.14).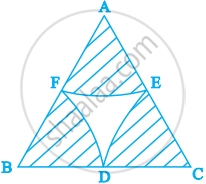
Three circles each of radius 3.5 cm are drawn in such a way that each of them touches the other two. Find the area enclosed between these circles.
Find the area of the sector of a circle of radius 5 cm, if the corresponding arc length is 3.5 cm.
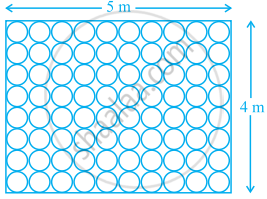
Floor of a room is of dimensions 5 m × 4 m and it is covered with circular tiles of diameters 50 cm each as shown in figure. Find the area of floor that remains uncovered with tiles. (Use π = 3.14)
The length of the minute hand of a clock is 6 cm. Find the area swept by it when it moves from 7:05 p.m. to 7:40 p.m.
