Advertisements
Advertisements
प्रश्न
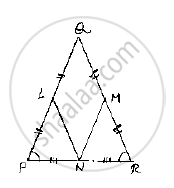
In a ΔPQR, if PQ = QR and L, M and N are the mid-points of the sides PQ, QR and RP
respectively. Prove that: LN = MN.
Advertisements
उत्तर
Given that, in PQR, PQ QRand L,M,N are midpoints of the sides PQ, QP and RP
respectively and given to prove that LN MN
Here we can observe that PQR is and isosceles triangle
⇒PQ =QR and ∠QPR =∠QRP ……..(1)
And also, L and M are midpoints of PQ and QR respectively
⇒ `PL=LQ=(PQ)/2,QM=MR=(QR)/2`
And also, PQ=QR
⇒ `PL=LQ=QM=MR=(PQ)/2=(QR)/2` .............(2)
Now, consider ΔLPN and ,Δ MRN
LP= MR [From – (2)]
∠LPN =∠MRN [From – (1)]
∵∠QPR and ∠LPN and ∠ QRP and ∠MRN are same
PN= NR [∵N is midpoint of PR]
So, by SAS congruence criterion, we have LPN≅ MRN
⇒LN =MN
[ ∵Corresponding parts of congruent triangles are equal]
APPEARS IN
संबंधित प्रश्न
In triangles ABC and PQR, if ∠A = ∠R, ∠B = ∠P and AB = RP, then which one of the following congruence conditions applies:
In an isosceles triangle, if the vertex angle is twice the sum of the base angles, then the measure of vertex angle of the triangle is
In the given figure, AB ⊥ BE and FE ⊥ BE. If BC = DE and AB = EF, then ΔABD is congruent to
ABC is an isosceles triangle such that AB = AC and AD is the median to base BC. Then, ∠BAD =
In the pair of triangles given below, the parts shown by identical marks are congruent. State the test and the one-to-one correspondence of vertices by which the triangles in the pair are congruent, the remaining congruent parts.
If the following pair of the triangle is congruent? state the condition of congruency :
In ΔABC and ΔDEF, ∠B = ∠E = 90o; AC = DF and BC = EF.
The following figure has shown a triangle ABC in which AB = AC. M is a point on AB and N is a point on AC such that BM = CN.
Prove that: (i) AM = AN (ii) ΔAMC ≅ ΔANB
A is any point in the angle PQR such that the perpendiculars drawn from A on PQ and QR are equal. Prove that ∠AQP = ∠AQR.
ΔABC is isosceles with AB = AC. BD and CE are two medians of the triangle. Prove that BD = CE.
The top and bottom faces of a kaleidoscope are congruent.
