Advertisements
Advertisements
प्रश्न
In the given figure, XAY is a tangent to the circle centered at O. If ∠ABO = 40°, then find ∠BAY and ∠AOB.

उत्तर

Given, ∠ABO = 40°
∠XAO = 90° ...(Angle between radius and tangent)
OA = OB ...(Radii of same circle)
⇒ ∠OAB = ∠OBA
∴ ∠OAB = 40°
Now, applying the linear pair of angles property,
we get
∠BAY + ∠OAB + ∠XAO = 180°
⇒ ∠BAY + 40° + 90° = 180°
⇒ ∠BAY + 130° = 180°
⇒ ∠BAY = 180° – 130°
⇒ ∠BAY = 50°
Now, In ΔAOB,
∠AOB + ∠OAB + ∠OBA = 180°
or, ∠AOB + 40° + 40° = 180°
or, ∠AOB = 180° – 80° = 100°
APPEARS IN
संबंधित प्रश्न
In triangle PQR, PQ = 24 cm, QR = –7 cm and ∠PQR = 90°. Find the radius of the inscribed circle.

Show that the circle drawn on any one of the equal sides of an isosceles triangle as diameter bisects the base.
If Δ ABC is isosceles with AB = AC and C (O, r) is the incircle of the ΔABC touching BC at L,prove that L bisects BC.
In fig., PT is a tangent to the circle at T and PAB is a secant to the same circle. If PB = 9cm and AB = 5 cm, find PT.
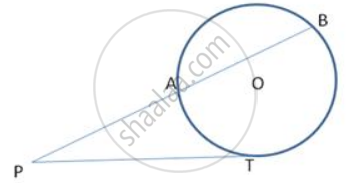
In the given figure, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD = 7.8 cm, PD = 5 cm, PB = 4 cm. Find AB.

In the given figure, O is the centre of the circle. Tangents at A and B meet at C. If angle ACO = 30°, find: angle AOB

In Fig. the incircle of ΔABC touches the sides BC, CA, and AB at D, E respectively. Show that: AF + BD + CE = AE + BF + CD = `1/2`( Perimeter of ΔABC)

In figure, M is the centre of the circle and seg KL is a tangent segment. If MK = 12, KL = `6sqrt(3)`, then find
(i) Radius of the circle.
(ii) Measures of ∠K and ∠M.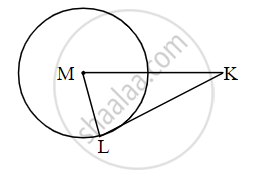
At one end A of a diameter AB of a circle of radius 5 cm, tangent XAY is drawn to the circle. The length of the chord CD parallel to XY and at a distance 8 cm from A is ______
Assertion (A): A tangent to a circle is perpendicular to the radius through the point of contact.
Reason (R): The lengths of tangents drawn from an external point to a circle are equal.
