Advertisements
Advertisements
प्रश्न
In figure, M is the centre of the circle and seg KL is a tangent segment. If MK = 12, KL = `6sqrt(3)`, then find
(i) Radius of the circle.
(ii) Measures of ∠K and ∠M.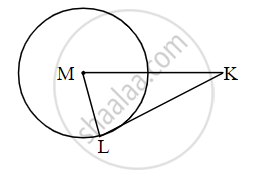
उत्तर
(i)
Line KL is the tangent to the circle at point L and seg ML is the radius. ......[Given]
∴ ∠MLK = 90° ......(i) [Tangent theorem]
In ∆MLK,
∠MLK = 90°
∴ MK2 = ML2 + KL2 .....[Pythagoras theorem]
∴ 122 = ML2 + `(6sqrt(3))^2`
∴ 144 = ML2 + 108
∴ ML2 = 144 − 108
∴ ML2 = 36
∴ ML = `sqrt(36)`
∴ ML = 6 units ......[Taking square root of both sides]
∴ Radius of the circle is 6 units.
(ii)
We know that,
ML = `1/2` MK,
∴ ∠K = 30° .....(ii) [Converse of 30°−60°−90° theorem]
In ∆MLK,
∠L = 90° .....[From (i)]
∠K = 30° .....[From (ii)]
∴ ∠M = 60° ......[Remaining angle of ∆MLK]
APPEARS IN
संबंधित प्रश्न
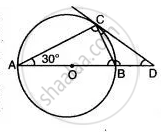
In Fig. 1, PQ is a tangent at a point C to a circle with centre O. if AB is a diameter and ∠CAB = 30°, find ∠PCA.

Draw a circle and two lines parallel to a given line such that one is a tangent and the other, a secant to the circle.
Four alternative answers for the following question is given. Choose the correct alternative.
If two circles are touching externally, how many common tangents of them can be drawn?
In the given Figure, AB and AC are tangents to the circle with centre O such that ∠BAC = 40°. Then ∠BOC is equal to ______

AB is a diameter and AC is a chord of a circle with centre O such that ∠BAC = 30°. The tangent at C intersects extended AB at a point D. Prove that BC = BD.
Find the length of the tangent from a point which is at a distance of 5cm from the centre of the circle of radius 3cm.
PA and PB are tangents from P to the circle with centre O. At M, a tangent is drawn cutting PA at K and PB at N. Prove that KN = AK + BN.
In the given figure, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD = 7.8 cm, PD = 5 cm, PB = 4 cm. Find the length of tangent PT.

In Figure, AB is diameter and AC is a chord of a circle such that ∠BAC = 30°. The tangent at C intersects AB produced at D. Prove that BC = BD.
If PA and PB are two tangents drawn from a point P to a circle with center C touching it A and B, prove that CP is the perpendicular bisector of AB.
In Fig. the incircle of ΔABC touches the sides BC, CA, and AB at D, E respectively. Show that: AF + BD + CE = AE + BF + CD = `1/2`( Perimeter of ΔABC)

In Fig. TA is a tangent to a circle from the point T and TBC is a secant to the circle. If AD is the bisector of ∠BAC, prove that ΔADT is isosceles.

Draw a circle of radius 2.7 cm and draw a chord PQ of length 4.5 cm. Draw tangents at points P and Q without using centre.
In figure, if ∠AOB = 125°, then ∠COD is equal to ______.

A: What is a line called, if it meets the circle at only one point?
B: Collection of all points equidistant from a fixed point is ______.
- Chord
- Tangent
- Circle
- Curve
- Secant
Which is correct matching?

In the above figure, seg AB and seg AD are tangent segments drawn to a circle with centre C from exterior point A, then prove that: ∠A = `1/2` [m(arc BYD) - m(arc BXD)]
The distance between two tangents parallel to each other of a circle is 13 cm. Find the radius of the circle.
In the given figure, PA is a tangent to the circle drawn from the external point P and PBC is the secant to the circle with BC as diameter. If ∠AOC = 130°, then find the measure of ∠APB, where O is the centre of the circle.

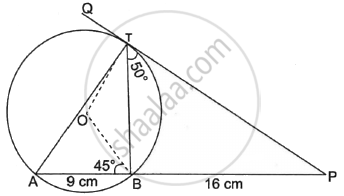
In the given figure, O is the centre of the circle. PQ is a tangent to the circle at T. Chord AB produced meets the tangent at P.
AB = 9 cm, BP = 16 cm, ∠PTB = 50° ∠OBA = 45°
Find:
- Length of PT
- ∠BAT
- ∠BOT
- ∠ABT
In the given figure O, is the centre of the circle. CE is a tangent to the circle at A. If ∠ABD = 26° find:
- ∠BDA
- ∠BAD
- ∠CAD
- ∠ODB

