Advertisements
Advertisements
प्रश्न
P and Q are respectively the mid-points of sides AB and BC of a triangle ABC and R is the mid-point of AP, show that
(i) ar(PRQ) = 1/2 ar(ARC)
(ii) ar(RQC) = 3/8 ar(ABC)
(iii) ar(PBQ) = ar(ARC)
उत्तर
Take a point S on AC such that S is the mid-point of AC.
Extend PQ to T such that PQ = QT.
Join TC, QS, PS, and AQ.
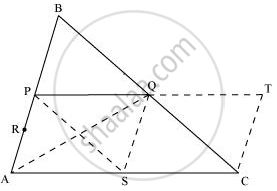
In ΔABC, P and Q are the mid-points of AB and BC respectively. Hence, by using mid-point theorem, we obtain
PQ || AC and PQ = 1/2AC
⇒ PQ || AS and PQ = AS (As S is the mid-point of AC)
∴ PQSA is a parallelogram. We know that diagonals of a parallelogram bisect it into equal areas of triangles.
∴ ar (ΔPAS) = ar (ΔSQP) = ar (ΔPAQ) = ar (ΔSQA)
Similarly, it can also be proved that quadrilaterals PSCQ, QSCT, and PSQB are also parallelograms and therefore,
ar (ΔPSQ) = ar (ΔCQS) (For parallelogram PSCQ)
ar (ΔQSC) = ar (ΔCTQ) (For parallelogram QSCT)
ar (ΔPSQ) = ar (ΔQBP) (For parallelogram PSQB)
Thus,
ar (ΔPAS) = ar (ΔSQP) = ar (ΔPAQ) = ar (ΔSQA) = ar (ΔQSC) = ar (ΔCTQ) = ar (ΔQBP) ... (1)
Also, ar (ΔABC) = ar (ΔPBQ) + ar (ΔPAS) + ar (ΔPQS) + ar (ΔQSC)
ar (ΔABC) = ar (ΔPBQ) + ar (ΔPBQ) + ar (ΔPBQ) + ar (ΔPBQ)
= 4 ar (ΔPBQ)
⇒ ar (ΔPBQ) = 1/4 ar(ΔABC) ... (2)
(i)Join point P to C.
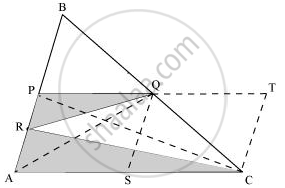
In ΔPAQ, QR is the median.
`therefore ar(trianglePRQ)=1/2ar(trianglePAQ)=1/2xx1/4ar(triangleABC)=1/8ar(triangleABC)" .........(3)"`
In ΔABC, P and Q are the mid-points of AB and BC respectively. Hence, by using mid-point theorem, we obtain
PQ = 1/2AC
AC = 2PQ ⇒ AC = PT
Also, PQ || AC ⇒ PT || AC
Hence, PACT is a parallelogram.
ar (PACT) = ar (PACQ) + ar (ΔQTC)
= ar (PACQ) + ar (ΔPBQ [Using equation (1)]
∴ ar (PACT) = ar (ΔABC) ... (4)
`ar(triangleARC) = 1/2ar(trianglePAC)" (CR is the median of ΔPAC)"`
`= 1/2xx1/2ar(PACT)" (PC is the diagonal parallelogram PACT)"`
`= 1/4 ar(PACT) = 1/4 ar(triangleABC)`
`rArr 1/2ar(triangleARC) = 1/8ar(triangleABC)`
`rArr1/2ar(triangleARC)=ar(trianglePRQ)" [Using equation (3)] .......(5)"`
(ii)
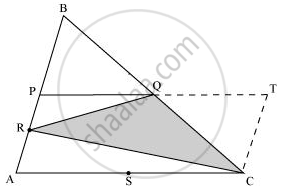
ar(PACT) = ar(ΔPRQ) + ar(ΔARC) + ar(ΔQTC) + ar(ΔRQC)
Putting the values from equations (1), (2), (3), (4), and (5), we obtain
`ar(ΔABC) = 1/8ar(ΔABC) + 1/4ar(ΔABC) + 1/4ar(ΔABC) + ar(ΔRQC)`
`ar(ΔABC) = 5/8ar(ΔABC) + ar(ΔRQC)`
`ar(ΔRQC) = (1-5/8)ar(ΔABC)`
`ar(ΔRQC) = 3/8ar(ΔABC)`
(iii) In parallelogram PACT,
`ar(ΔARC) = 1/2ar(ΔPAC)" (CR is the median of ΔPAC)"`
`= 1/2xx1/2ar(PACT)" (PC is the diagonal of parallelogram PACT)"`
`= 1/4ar(PACT)`
`= 1/4ar(ABC)`
= ar(ΔPBQ)
APPEARS IN
संबंधित प्रश्न
In the given figure, diagonals AC and BD of quadrilateral ABCD intersect at O such that OB = OD. If AB = CD, then show that:
(i) ar (DOC) = ar (AOB)
(ii) ar (DCB) = ar (ACB)
(iii) DA || CB or ABCD is a parallelogram.
[Hint: From D and B, draw perpendiculars to AC.]

D and E are points on sides AB and AC respectively of ΔABC such that
ar (DBC) = ar (EBC). Prove that DE || BC.
ABCD is a trapezium with AB || DC. A line parallel to AC intersects AB at X and BC at Y. Prove that ar (ADX) = ar (ACY).
[Hint: Join CX.]
In the given figure, AP || BQ || CR. Prove that ar (AQC) = ar (PBR).

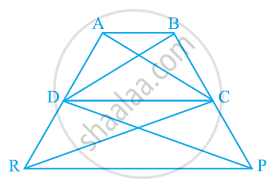
In the given figure, ar (DRC) = ar (DPC) and ar (BDP) = ar (ARC). Show that both the quadrilaterals ABCD and DCPR are trapeziums.
In Fig. below, ABC and BDE are two equilateral triangles such that D is the mid-point of
BC. AE intersects BC in F. Prove that
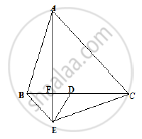
(1) ar (Δ BDE) = `1/2` ar (ΔABC)
(2) Area ( ΔBDE) `= 1/2 ` ar (ΔBAE)
(3) ar (BEF) = ar (ΔAFD)
(4) area (Δ ABC) = 2 area (ΔBEC)
(5) ar (ΔFED) `= 1/8` ar (ΔAFC)
(6) ar (Δ BFE) = 2 ar (ΔEFD)
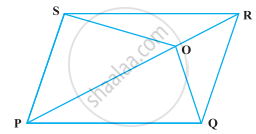
PQRS is a parallelogram whose area is 180 cm2 and A is any point on the diagonal QS. The area of ∆ASR = 90 cm2.
The area of the parallelogram ABCD is 90 cm2 (see figure). Find ar (ΔABD)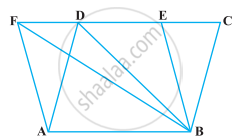
O is any point on the diagonal PR of a parallelogram PQRS (Figure). Prove that ar (PSO) = ar (PQO).
In the following figure, ABCDE is any pentagon. BP drawn parallel to AC meets DC produced at P and EQ drawn parallel to AD meets CD produced at Q. Prove that ar (ABCDE) = ar (APQ)