Advertisements
Advertisements
प्रश्न
Prove the following: `(tan(90° - θ)cotθ)/("cosec"^2 θ)` = cos2θ
उत्तर
L.H.S.
= `(tan(90° - θ)cotθ)/("cosec"^2 θ)`
= `(cotθ xx cotθ)/("cosec^2 θ)`
= `(cot^2 θ)/("cosec"^2 θ)`
= `((cos^2 θ)/(sin^2 θ))/((1)/(sin^2 θ)`
= cos2θ
= R.H.S.
APPEARS IN
संबंधित प्रश्न
If 2 cos 2A = `sqrt3` and A is acute,
find:
(i) A
(ii) sin 3A
(iii) sin2 (75° - A) + cos2 (45° +A)
Calculate the value of A, if (tan A - 1) (cosec 3A - 1) = 0
Calculate the value of A, if (cosec 2A - 2) (cot 3A - 1) = 0
If tanθ= cotθ and 0°≤ θ ≤ 90°, find the value of 'θ'.
In the given figure, AB and EC are parallel to each other. Sides AD and BC are 1.5 cm each and are perpendicular to AB. Given that ∠AED = 45° and ∠ACD = 30°. Find:
a. AB
b. AC
c. AE
Find:
a. BC
b. AD
c. AC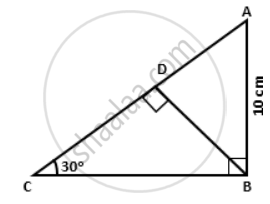
Find the value 'x', if: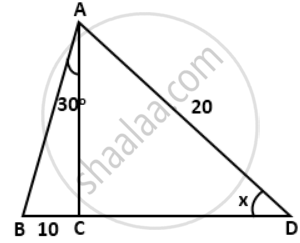
In the given figure; ∠B = 90°, ∠ADB = 30°, ∠ACB = 45° and AB = 24 m. Find the length of CD.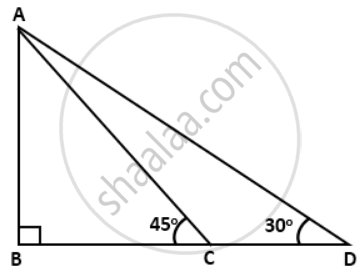
Express each of the following in terms of trigonometric ratios of angles between 0° and 45°: tan77° - cot63° + sin57°
If sin(θ - 15°) = cos(θ - 25°), find the value of θ if (θ-15°) and (θ - 25°) are acute angles.
