Advertisements
Advertisements
प्रश्न
Two concentric circles of radii a and b (a > b) are given. Find the length of the chord of the larger circle which touches the smaller circle.
उत्तर

Let the two concentric circles be centered at point O.
Also, a > b where a is the radius of a larger circle and b is the radius of the smaller circle.
And let PQ be the chord of the larger circle which touches the smaller circle at point A. Therefore, PQ is tangent to the smaller circle.
OA ⊥ PQ (As OA is the radius of the circle)
Applying Pythagoras theorem in ΔOAP, we obtain
OA2+AP2=OP2
b2 + PA2 = a2
⇒ PA2 = a2 - b2
⇒ PA = `sqrt("a"^2-"b"^2)`
In ΔOPQ,
Since OA ⊥ PQ,
PA = AQ (Perpendicular from the center of the circle to the chord bisects the chord)
∴ PQ =2PA = `2sqrt("a"^2-"b"^2)`
Therefore, the length of the chord of the larger circle is `2sqrt("a"^2-"b"^2)` cm
APPEARS IN
संबंधित प्रश्न
Prove that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
Prove that a parallelogram circumscribing a circle is a rhombus.
In the given figure, AD is a diameter. O is the centre of the circle. AD is parallel to BC and ∠CBD = 32°.
Find:
- ∠OBD
- ∠AOB
- ∠BED

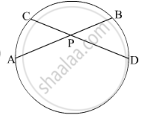
In the given figure PA = 10, PB = 2 and PC = 5. Find PD.
Prove that the lengths of two tangent segments drawn to the circle from an external point are equal.
Construct a pair of tangents to a circle of radius 4 cm from a point which is at a distance of 6 cm from its centre.
In a circle of radius 17 cm, two parallel chords are drawn on opposite sides of a diameter. The distance between the chords is 23 cm. If the length of one chord is 16 cm, then the length of the other is ______
Two tangents PQ and PR are drawn from an external point to a circle with centre O. Prove that QORP is a cyclic quadrilateral.
From an external point P, two tangents, PA and PB are drawn to a circle with centre O. At one point E on the circle tangent is drawn which intersects PA and PB at C and D, respectively. If PA = 10 cm, find the the perimeter of the triangle PCD.
A quadrilateral PQRS is drawn to circumscribe a circle. If PQ = 12 cm, QR = 15 cm and RS = 14 cm, then find the length of SP is ______.