Question

Two isosceles triangles have equal angles and their areas are in the ratio 16 : 25. The ratio of their corresponding heights is 

पर्याय

• 4 : 5

• 5 : 4

• 3 : 2

• 5 : 7

Answer 1

Given: Two isosceles triangles have equal vertical angles and their areas are in the ratio of 16:25.

To find: Ratio of their corresponding heights.

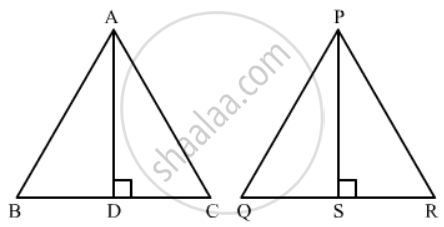
Let ∆ABC and ∆PQR be two isosceles triangles such that

\[\angle A = \angle P\] . Suppose AD ⊥ BC and PS ⊥ QR .

In ∆ABC and ∆PQR, 

\[\frac{AB}{PQ} = \frac{AC}{PR}\]
\[\angle A = \angle P\]
\[ \therefore ∆ ABC~ ∆ PQR \left( SAS similarity \right)\]

We know that the ratio of areas of two similar triangles is equal to the ratio of squares of their corresponding altitudes.

Hence,

\[\frac{Ar\left( ∆ ABC \right)}{Ar\left( ∆ PQR \right)} = \left( \frac{AD}{PS} \right)^2 \]
\[ \Rightarrow \frac{16}{25} = \left( \frac{AD}{PS} \right)^2 \]
\[ \Rightarrow \frac{AD}{PS} = \frac{4}{5}\]

Hence we got the result as `a`