Advertisements
Advertisements
प्रश्न
Use the given figure to find:
(i) tan θ°
(ii) θ°
(iii) sin2θ° - cos2θ°
(iv) Use sin θ° to find the value of x.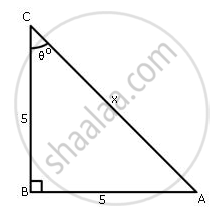
उत्तर
(i) tan θ° = `(5)/(5) = 1`
(ii) tan θ° = 1
tan θ° = tan 45°
θ° = 45°
(iii) sin2θ° – cos2θ° = sin245° – cos2 45°
= `(1/sqrt2)^2 – (1/sqrt2)^2`
= 0
(iv) sinθ° = `(5)/(x)`
sin 45° = `(5)/(x)`
x = `(5)/(sin45°)`
x = `(5)/(1/sqrt2)`
x = 5`sqrt2`
APPEARS IN
संबंधित प्रश्न
Calculate the value of A, if (sin A - 1) (2 cos A - 1) = 0
Solve for 'θ': `sec(θ/2 + 10°) = (2)/sqrt(3)`
If tanθ= cotθ and 0°≤ θ ≤ 90°, find the value of 'θ'.
If θ = 30°, verify that: sin2θ = `(2tanθ)/(1 ++ tan^2θ)`
Find the value of 'y' if `sqrt(3)` = 1.723.
Given your answer correct to 2 decimal places.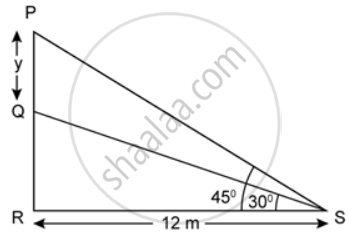
In right-angled triangle ABC; ∠B = 90°. Find the magnitude of angle A, if:
a. AB is `sqrt(3)` times of BC.
B. BC is `sqrt(3)` times of BC.
Evaluate the following: `(sin62°)/(cos28°)`
Evaluate the following: cosec 54° - sec 36°
Evaluate the following: sin22° cos44° - sin46° cos68°
Evaluate the following: `(3sin37°)/(cos53°) - (5"cosec"39°)/(sec51°) + (4tan23° tan37° tan67° tan53°)/(cos17° cos67° "cosec"73° "cosec"23°)`
