Advertisements
Advertisements
Question
A 100 kg lock is started with a speed of 2.0 m s−1 on a long, rough belt kept fixed in a horizontal position. The coefficient of kinetic friction between the block and the belt is 0.20. (a) Calculate the change in the internal energy of the block-belt system as the block comes to a stop on the belt. (b) Consider the situation from a frame of reference moving at 2.0 m s−1 along the initial velocity of the block. As seen from this frame, the block is gently put on a moving belt and in due time the block starts moving with the belt at 2.0 m s−1. calculate the increase in the kinetic energy of the block as it stops slipping past the belt. (c) Find the work done in this frame by the external force holding the belt.
Solution
Here,
m = 100 kg
u = 2.0 m/s
v = 0
μk = 0.2
a) Internal energy of the belt-block system will decrease when the block will lose its KE in heat due to friction. Thus,
KE lost = `1/2m u^2-1/2mnu^2`
`1/2m(u^2-nu^2)`
`1/2xx100xx(2^2-0^2)`
= 200 J
b) Velocity of the frame is given by
uf = 2.0 m/s
u’ = u - uf = 2 – 2= 0
v’ = 0 – 2 = -2 m/s
KE lost = `1/2m u'^2-1/2mnu'^2`
`=1/2m(0^2-nu'^2)`
`=1/2xx100xx(0^2-2^2)`
= 200 J
c) Force of friction is given by
`f=mu_k^""R`
`rArrf=0.2xxmg=0.2xx100xx10=200N
`"Retardation"=f/m=200/100=2ms^-2`
Distance moved by the block will be as seen from the frame = s
`nu'^2-u'^2=2as`
`rArr2^2-0^2=2xx2s`
`rArrs=1"m"`
Work done by the force responsible for accelaration as seen from the frame = fs
= 200 x 1 = 200J
Work done by the belt to give it a final velocity of 2 m/s
`=1/2mnu'^2`
`=1/2xx100xx(2)^2`
= 200J
Total work done by external force as seen from the frame 200 + 200 = 400J
APPEARS IN
RELATED QUESTIONS
Should the internal energy of a system necessarily increase if heat is added to it?
A cylinder containing a gas is lifted from the first floor to the second floor. What is the amount of work done on the gas? What is the amount of work done by the gas? Is the internal energy of the gas increased? Is the temperature of the gas increased?
A force F is applied on a block of mass M. The block is displaced through a distance d in the direction of the force. What is the work done by the force on the block? Does the internal energy change because of this work?
A closed bottle contains some liquid. the bottle is shaken vigorously for 5 minutes. It is found that the temperature of the liquid is increased. Is heat transferred to the liquid? Is work done on the liquid? Neglect expansion on heating.
The final volume of a system is equal to the initial volume in a certain process. Is the work done by the system necessarily zero? Is it necessarily nonzero?
Can work be done by a system without changing its volume?
An ideal gas is pumped into a rigid container having diathermic walls so that the temperature remains constant. In a certain time interval, the pressure in the container is doubled. Is the internal energy of the contents of the container also doubled in the interval ?
When a tyre bursts, the air coming out is cooler than the surrounding air. Explain.
Figure shows two processes A and B on a system. Let ∆Q1 and ∆Q2 be the heat given to the system in processes A and B respectively. Then ____________ .

A gas is contained in a metallic cylinder fitted with a piston. The piston is suddenly moved in to compress the gas and is maintained at this position. As time passes the pressure of the gas in the cylinder ______________ .
A gas is initially at a pressure of 100 kPa and its volume is 2.0 m3. Its pressure is kept constant and the volume is changed from 2.0 m3 to 2.5 m3. Its Volume is now kept constant and the pressure is increased from 100 kPa to 200 kPa. The gas is brought back to its initial state, the pressure varying linearly with its volume. (a) Whether the heat is supplied to or extracted from the gas in the complete cycle? (b) How much heat was supplied or extracted?
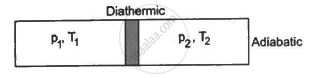
Figure shows a cylindrical tube of volume V with adiabatic walls containing an ideal gas. The internal energy of this ideal gas is given by 1.5 nRT. The tube is divided into two equal parts by a fixed diathermic wall. Initially, the pressure and the temperature are p1, T1 on the left and p2, T2 on the right. The system is left for sufficient time so that the temperature becomes equal on the two sides. (a) How much work has been done by the gas on the left part? (b) Find the final pressures on the two sides. (c) Find the final equilibrium temperature. (d) How much heat has flown from the gas on the right to the gas on the left?
Which of the following system freely allows the exchange of energy and matter with its environment?
A system releases 100 kJ of heat while 80 kJ of work is done on the system. Calculate the change in internal energy.
One gram of water (1 cm3) becomes 1671 cm3 of steam at a pressure of 1 atm. The latent heat of vaporization at this pressure is 2256 J/g. Calculate the external work and the increase in internal energy.
Which of the following represents isothermal process?
n mole of a perfect gas undergoes a cyclic process ABCA (see figure) consisting of the following processes:
A `→` B: Isothermal expansion at temperature T so that the volume is doubled from V1 to V2 = 2V1 and pressure changes from P1 to P2.
B `→` C: Isobaric compression at pressure P2 to initial volume V1.
C `→` A: Isochoric change leading to change of pressure from P2 to P1.
Total workdone in the complete cycle ABCA is ______.

In thermodynamics, heat and work are ______.
The internal energy of one mole of argon is ______.
