Advertisements
Advertisements
Question
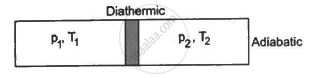
Figure shows a cylindrical tube of volume V with adiabatic walls containing an ideal gas. The internal energy of this ideal gas is given by 1.5 nRT. The tube is divided into two equal parts by a fixed diathermic wall. Initially, the pressure and the temperature are p1, T1 on the left and p2, T2 on the right. The system is left for sufficient time so that the temperature becomes equal on the two sides. (a) How much work has been done by the gas on the left part? (b) Find the final pressures on the two sides. (c) Find the final equilibrium temperature. (d) How much heat has flown from the gas on the right to the gas on the left?
Advertisements
Solution
Let n1 , U1 and n2 ,U2 be the no. of moles , internal energy of ideal gas in the left chamber and right chamber respectively.
(a) As the diathermic wall is fixed, so final volume of the chambers will be same. Thus, ΔV = 0, hence work done ΔW= P Δ V = 0
by eq. of state in the first and second chamber
`P_1V/2=n_1RT_1`
`rArr n_1=(P_1V)/(2RT_1)`
`P_2V/2=n_2RT_2`
`rArr n_2=(P_2V)/(2RT_2)`
n = n1 + n2
`rArrn=(P_1V)/(2RT_1)+(P_2V)/(2RT_2)=V/(2R)((P_1T_2+P_2T_1)/(T_1T_2))`
Again,
U = nCvT
`rArrnC_"v"T=1.5nRT`
`rArrC_"v"=1.5R`
In the first and second chamber internal energy is given by
U1 = n1CvT1 = n1 1.5RT1
U2 = n2CvT2 = n2 1.5RT2
U = U1 + U2
1.5nRT = n1 1.5RT1 + n2 1.5RT2
⇒ nT = n1T1 + n2T2
`rArrnT = (P_1V)/(2RT_1)T_1+(P-2V)/(2RT_2)T_2=((P_1+P_2)V)/(2R)`
`rArrT=((P_1+P_2)V)/(2nR)=((P_1+P_2)V)/(2RV/(2R)((P_1T_2+P_2T_1)/(T_1T_2)))=(T_1T_2(P_1+P_2))/(P_1T_2+P_2T_1)................(1)`
b) Let final pressure in the first and second compartment P1' and P2'.
By five variable equ of state in the first chamber
`(P_1V/2)/T_1=(P_1'V/2)/T`
`rArrP_1'=P_1/T_1T`
By eq. (1)
`rArrP_1'=P_1/T_1(T_1T_2(P_1+P_2))/(P_1T_2+P_2T_1)=(P_1T_2(P_1+P_2))/(P_1T_2+P_2T_1)`
Similarly,
`rArrP_1'=P_2/T_2T=(P_2T_1(P_1+P_2))/(P_1T_2+P_2T_1)`
c) Final temperature will be
`T=(T_1T_2(P_1+P_2))/(P_1T_2+P_2T_1).............\left(\text{by equation (1)}\right)`
d) Heat lost by right chamber will be
n2CvT2 - n2CvT
`=(P_2V)/(2RT_2)1.5RT_2-(P_2V)/(2RT_2)1.5R(T_1T_2(P_1+P_2))/(P_1T_2+P_2T_1)`
`=(3P_2V)/4-(3P_2V)/4(T_1(P_1+P_2))/(P_1T_2+P_2T_1)`
`=(3P_2V)/4[1-(T_1(P_1+P_2))/(P_1T_2+P_2T_1)]`
`=(3P_2V)/4[(P_1T_2+P_2T_1-T_1(P_1+P_2))/(P_1T_2+P_2T_1)]`
`=(3P_1P_2V)/4[(T_2-T_1)/(P_1T_2+P_2T_1)]`
APPEARS IN
RELATED QUESTIONS
Explain why Two bodies at different temperatures T1 and T2, if brought in thermal contact, do not necessarily settle to the mean temperature (T1 + T2)/2.
The outer surface of a cylinder containing a gas is rubbed vigorously by a polishing machine. The cylinder and its gas become warm. Is the energy transferred to the gas heat or work?
Consider the process on a system shown in figure. During the process, the work done by the system ______________ .
Consider the following two statements.
(A) If heat is added to a system, its temperature must increase.
(B) If positive work is done by a system in a thermodynamic process, its volume must increase.
In a process on a system, the initial pressure and volume are equal to the final pressure and volume.
(a) The initial temperature must be equal to the final temperature.
(b) The initial internal energy must be equal to the final internal energy.
(c) The net heat given to the system in the process must be zero.
(d) The net work done by the system in the process must be zero.
A gas is initially at a pressure of 100 kPa and its volume is 2.0 m3. Its pressure is kept constant and the volume is changed from 2.0 m3 to 2.5 m3. Its Volume is now kept constant and the pressure is increased from 100 kPa to 200 kPa. The gas is brought back to its initial state, the pressure varying linearly with its volume. (a) Whether the heat is supplied to or extracted from the gas in the complete cycle? (b) How much heat was supplied or extracted?
Which of the following system freely allows the exchange of energy and matter with its environment?
Define heat.
An ideal gas is compressed at a constant temperature. Its internal energy will ____________.
8 m3 of a gas is heated at the pressure 105 N/m2 until its volume increases by 10%. Then, the external work done by the gas is ____________.
Two samples A and B, of a gas at the same initial temperature and pressure are compressed from volume V to V/2; A isothermally and B adiabatically. The final pressure of A will be ______.
Which of the following represents isothermal process?
Two cylinders A and B of equal capacity are connected to each other via a stopcock. A contains a gas at standard temperature and pressure. B is completely evacuated. The entire system is thermally insulated. The stopcock is suddenly opened. Answer the following:
What is the change in internal energy of the gas?
Figure shows the P-V diagram of an ideal gas undergoing a change of state from A to B. Four different parts I, II, III and IV as shown in the figure may lead to the same change of state.
- Change in internal energy is same in IV and III cases, but not in I and II.
- Change in internal energy is same in all the four cases.
- Work done is maximum in case I
- Work done is minimum in case II.
A person of mass 60 kg wants to lose 5kg by going up and down a 10 m high stairs. Assume he burns twice as much fat while going up than coming down. If 1 kg of fat is burnt on expending 7000 kilo calories, how many times must he go up and down to reduce his weight by 5 kg?
A gas is compressed at a constant pressure of 50 N/m2 from a volume of 10 m3 to a volume of 4 m3. Energy of 100 J is then added to the gas by heating. Its internal energy is ______.
If a gas is compressed adiabatically:
The molar specific heat of He at constant volume is 12.47 J/mol.K. Two moles of He are heated at constant pressure. So the rise in temperature is 10 K. Find the increase in internal energy of the gas.
What is heat?
