Advertisements
Advertisements
Question
A radioactive isotope is being produced at a constant rate dN/dt = R in an experiment. The isotope has a half-life t1/2. Show that after a time t >> t1/2 the number of active nuclei will become constant. Find the value of this constant.
Solution
Given:
Half life period of isotope = t1/2
Disintegration constant, `lambda = 0.693/t_"1/2"`
Rate of Radio active decay (R) is given by
`R = "dN"/"dt"`
We are to show that after time t >> `t_"1/2"`the number of active nuclei is constant.
`("dN"/"dt")_"present" = R = ("dN"/"dt")_"decay"`
`therefore R = ("dN"/"dt")_"decay"`
Rate of radioactive decay, `R = lambdaN`
Here, λ = Radioactive decay constant
N = Constant number
`R = 0.693/t_"1/2" xx N`
⇒`Rt_"1/2" = 0.693 N`
⇒`N = (Rt_"1/2")/0.693`
This value of N should be constant.
APPEARS IN
RELATED QUESTIONS
Derive the mathematical expression for law of radioactive decay for a sample of a radioactive nucleus
Under certain circumstances, a nucleus can decay by emitting a particle more massive than an α-particle. Consider the following decay processes:
\[\ce{^223_88Ra -> ^209_82Pb + ^14_6C}\]
\[\ce{^223_88 Ra -> ^219_86 Rn + ^4_2He}\]
Calculate the Q-values for these decays and determine that both are energetically allowed.
(a) Derive the relation between the decay constant and half life of a radioactive substance.
(b) A radioactive element reduces to 25% of its initial mass in 1000 years. Find its half life.
Define the activity of a given radioactive substance. Write its S.I. unit.
A freshly prepared radioactive source of half-life 2 h emits radiation of intensity which is 64 times the permissible safe level. The minimum time after which it would be possible to work safely with this source is
The decay constant of a radioactive sample is λ. The half-life and the average-life of the sample are respectively
Consider the situation of the previous problem. Suppose the production of the radioactive isotope starts at t = 0. Find the number of active nuclei at time t.
The half-life of 40K is 1.30 × 109 y. A sample of 1.00 g of pure KCI gives 160 counts s−1. Calculate the relative abundance of 40K (fraction of 40K present) in natural potassium.
Define the term 'decay constant' of a radioactive sample. The rate of disintegration of a given radioactive nucleus is 10000 disintegrations/s and 5,000 disintegrations/s after 20 hr. and 30 hr. respectively from start. Calculate the half-life and the initial number of nuclei at t= 0.
Disintegration rate of a sample is 1010 per hour at 20 hours from the start. It reduces to 6.3 x 109 per hour after 30 hours. Calculate its half-life and the initial number of radioactive atoms in the sample.
Obtain an expression for the decay law of radioactivity. Hence show that the activity A(t) =λNO e-λt.
A radioactive element disintegrates for an interval of time equal to its mean lifetime. The fraction that has disintegrated is ______
Which one of the following nuclei has shorter meant life?
The half-life of a radioactive sample undergoing `alpha` - decay is 1.4 x 1017 s. If the number of nuclei in the sample is 2.0 x 1021, the activity of the sample is nearly ____________.
If 10% of a radioactive material decay in 5 days, then the amount of original material left after 20 days is approximately :
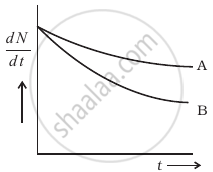
The variation of decay rate of two radioactive samples A and B with time is shown in figure.

Which of the following statements are true?
- Decay constant of A is greater than that of B, hence A always decays faster than B.
- Decay constant of B is greater than that of A but its decay rate is always smaller than that of A.
- Decay constant of A is greater than that of B but it does not always decay faster than B.
- Decay constant of B is smaller than that of A but still its decay rate becomes equal to that of A at a later instant.
Which sample, A or B shown in figure has shorter mean-life?
The activity R of an unknown radioactive nuclide is measured at hourly intervals. The results found are tabulated as follows:
| t (h) | 0 | 1 | 2 | 3 | 4 |
| R (MBq) | 100 | 35.36 | 12.51 | 4.42 | 1.56 |
- Plot the graph of R versus t and calculate the half-life from the graph.
- Plot the graph of ln `(R/R_0)` versus t and obtain the value of half-life from the graph.
The radioactivity of an old sample of whisky due to tritium (half-life 12.5 years) was found to be only about 4% of that measured in a recently purchased bottle marked 10 years old. The age of a sample is ______ years.
