Advertisements
Advertisements
Question
A six sided die is marked ‘1’ on one face, ‘3’ on two of its faces, and ‘5’ on remaining three faces. The die is thrown twice. If X denotes the total score in two throws, find the cumulative distribution function
Solution
Let X be the random variable denotes the total score in two thrown of a die.
Sample space S
| I\II | 1 | 3 | 3 | 5 | 5 | 5 |
| 1 | 2 | 4 | 4 | 6 | 6 | 6 |
| 3 | 4 | 6 | 6 | 8 | 8 | 8 |
| 3 | 4 | 6 | 6 | 8 | 8 | 8 |
| 5 | 6 | 8 | 8 | 10 | 10 | 10 |
| 5 | 6 | 8 | 8 | 10 | 10 | 10 |
| 5 | 6 | 8 | 8 | 10 | 10 | 10 |
n(S) = 36
X = {2, 4, 6, 8, 10}
| Values of the random variable | 2 | 4 | 6 | 8 | 10 | Total |
| Number of elements in inverse image | 1 | 4 | 10 | 12 | 9 | 36 |
Cumulative distribution function
F(x) = P(X ≤ x)
= `sum_(x_"i" ≤ x) "P"("X" = x_"i")`
F(2) = P(X < 2)
= P(X < 2) + P(X = 2)
= `0 + 1/36`
= `1/36`
F(4) = `"P"("X" ≤ 4)`
= P(X <2) + P(X = 2) + P(X = 4)
= `0+ 1/36 + 4/36`
= `5/36`
F(6) = `"P"("X" ≤ 6)`
= P(X < 2) + P(X = 2) + P(X = 4) + P(X = 6)
= `0 + 11/36+ 4/36 + 10/36`
= `15/36`
F(8) = P(X ≤ 8)
= P(X < 2) + P(X = 2) + P(X = 4) + P(X = 6) + P(X = 8)
= `0 + 1/6 + 4/36 + 10/36 + 12/36`
= `27/36`
F(10) = P(X ≤ 10)
= P(X < 2) + P(X = 2) + P(x = 8) + P(X = 10)
= `0 + 1/36 + 4/36 + 10/36 + 12/36 + 9/36`
= `36/36`
= 1
F(x) = `{{:(0",", "For" - oo < x < 2),(1/36",", "For" 2 ≤ x ≤ 4),(5/36",", "For" 4 ≤ x < 6),(15/36",", "For" 6 ≤ x < 8),(27/36",", "For" 8 ≤ x < 10),(1",", "For" 10 ≤ x < oo):}`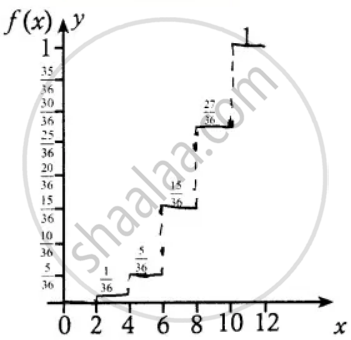
APPEARS IN
RELATED QUESTIONS
Suppose error involved in making a certain measurement is continuous r.v. X with p.d.f.
f (x) = k `(4 – x^2 )`, for –2 ≤ x ≤ 2 and = 0 otherwise.
P(x > 0)
Suppose error involved in making a certain measurement is continuous r.v. X with p.d.f.
`"f(x)" = {("k"(4 - x^2) "for –2 ≤ x ≤ 2,"),(0 "otherwise".):}`
P(–1 < x < 1)
The following is the p.d.f. of continuous r.v.
f (x) = `x/8`, for 0 < x < 4 and = 0 otherwise.
Find expression for c.d.f. of X
Given the p.d.f. of a continuous r.v. X ,
f (x) = `x^2/3` , for –1 < x < 2 and = 0 otherwise
Determine c.d.f. of X hence find P(1 < x < 2)
It is felt that error in measurement of reaction temperature (in celsius) in an experiment is a continuous r.v. with p.d.f.
f(x) = `{(x^3/(64), "for" 0 ≤ x ≤ 4),(0, "otherwise."):}`
Find P(0 < X ≤ 1).
It is felt that error in measurement of reaction temperature (in celsius) in an experiment is a continuous r.v. with p.d.f.
f(x) = `{(x^3/(64), "for" 0 ≤ x ≤ 4),(0, "otherwise."):}`
Find probability that X is between 1 and 3..
F(x) is c.d.f. of discrete r.v. X whose p.m.f. is given by P(x) = `"k"^4C_x` , for x = 0, 1, 2, 3, 4 and P(x) = 0 otherwise then F(5) = _______
Three fair coins are tossed simultaneously. Find the probability mass function for a number of heads that occurred
A six sided die is marked ‘1’ on one face, ‘3’ on two of its faces, and ‘5’ on remaining three faces. The die is thrown twice. If X denotes the total score in two throws, find P(4 ≤ X < 10)
The cumulative distribution function of a discrete random variable is given by
F(x) = `{{:(0, - oo < x < - 1),(0.15, - 1 ≤ x < 0),(0.35, 0 ≤ x < 1),(0.60, 1 ≤ x < 2),(0.85, 2 ≤ x < 3),(1, 3 ≤ x < oo):}`
Find P(X < 1)
The cumulative distribution function of a discrete random variable is given by
F(x) = `{{:(0, - oo < x < - 1),(0.15, - 1 ≤ x < 0),(0.35, 0 ≤ x < 1),(0.60, 1 ≤ x < 2),(0.85, 2 ≤ x < 3),(1, 3 ≤ x < oo):}`
Find P(X ≥ 2)
A random variable X has the following probability mass function.
| x | 1 | 2 | 3 | 4 | 5 |
| F(x) | k2 | 2k2 | 3k2 | 2k | 3k |
Find P(X > 3)
The cumulative distribution function of a discrete random variable is given by
F(x) = `{{:(0, "for" - oo < x < 0),(1/2, "for" 0 ≤ x < 1),(3/5, "for" 1 ≤ x < 2),(4/5, "for" 2 ≤ x < 4),(9/5, "for" 3 ≤ x < 4),(1, "for" ≤ x < oo):}`
Find P(X < 3)
Let X = time (in minutes) that lapses between the ringing of the bell at the end of a lecture and the actual time when the professor ends the lecture. Suppose X has p.d.f.
f(x) = `{(kx^2"," 0 ≤ x ≤ 2), (0"," "othenwise"):}`
Then, the probability that the lecture ends within 1 minute of the bell ringing is ______
The p.m.f. of a random variable X is
P(x) = `(5 - x)/10`, x = 1, 2, 3, 4
= 0, otherwise
The value of E(X) is ______
If the probability function of a random variable X is defined by P(X = k) = a`((k + 1)/2^k)` for k - 0, 1, 2, 3, 4, 5, then the probability that X takes a prime value is ______
The c.d.f. of a discrete r.v. X is
| X = x | -4 | -2 | -1 | 0 | 2 | 4 | 6 | 8 |
| F(x) | 0.2 | 0.4 | 0.55 | 0.6 | 0.75 | 0.80 | 0.95 | 1 |
Then P(X ≤ 4|X > -1) = ?
The p.d.f. of a continuous random variable X is
f(x) = 0.1 x, 0 < x < 5
= 0, otherwise
Then the value of P(X > 3) is ______
A random variable X has the following probability distribution:
| X = xi | 1 | 2 | 3 | 4 |
| P(X = xi) | 0.2 | 0.15 | 0.3 | 0.35 |
The mean and the variance are respectively ______.
For the following distribution function F(x) of a rv.x.
| x | 1 | 2 | 3 | 4 | 5 | 6 |
| F(x) | 0.2 | 0.37 | 0.48 | 0.62 | 0.85 | 1 |
P(3 < x < 5) =
