Advertisements
Advertisements
Question
A small compass needle of magnetic moment ‘m’ is free to turn about an axis perpendicular to the direction of uniform magnetic field ‘B’. The moment of inertia of the needle about the axis is ‘I’. The needle is slightly disturbed from its stable position and then released. Prove that it executes simple harmonic motion. Hence deduce the expression for its time period.
Solution
The torque on the needle is τ = m × B
In magnitude τ = mB sinθ
Here τ is restoring torque and θ is the angle between m and B.
Therefore, in equilibrium `I (d^2θ)/dt^2 =-mBsinθ`
Negative sign with mB sinθ implies that restoring torque is in opposition to deflecting torque. For small values of θ in radians, we approximate sinθ= θ and get
`I (d^2θ)/dt^2 =-mBθ`
or
`(d^2θ)/dt^2 =-(mB)/Iθ`
This represents a simple harmonic motion. The square of the angular frequency is ω2 = mB/J and the time period is,`T = 2πsqrtI/(mB)`
APPEARS IN
RELATED QUESTIONS
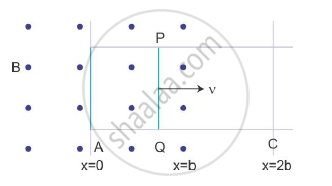
Sketch the change in flux, emf and force when a conducting rod PQ of resistance R and length l moves freely to and fro between A and C with speed v on a rectangular conductor placed in uniform magnetic field as shown in the figure
Can a charged particle be accelerated by a magnetic field? Can its speed be increased?
A particle moves in a region with a uniform magnetic field and a parallel, uniform electric field. At some instant, the velocity of the particle is perpendicular to the field direction. The path of the particle will be
Consider a solid sphere of radius r and mass m that has a charge q distributed uniformly over its volume. The sphere is rotated about its diameter with an angular speed ω. Show that the magnetic moment µ and the angular momentum l of the sphere are related as `mu = q/(2m) l`
Consider a straight piece of length x of a wire carrying a current i. Let P be a point on the perpendicular bisector of the piece, situated at a distance d from its middle point. Show that for d >> x, the magnetic field at P varies as 1/d2 whereas for d << x, it varies as 1/d.
A long, straight wire is fixed horizontally and carries a current of 50.0 A. A second wire having linear mass density 1.0 × 10−4 kg m−1 is placed parallel to and directly above this wire at a separation of 5.0 mm. What current should this second wire carry such that the magnetic repulsion can balance its weight?
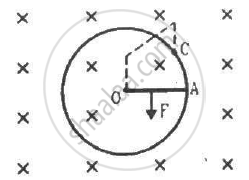
Consider the situation shown in the figure. Suppose the circular loop lies in a vertical plane. The rod has a mass m. The rod and the loop have negligible resistances but the wire connecting O and C has a resistance R. The rod is made to rotate with a uniform angular velocity ω in the clockwise direction by applying a force at the midpoint of OA in a direction perpendicular to it. Find the magnitude of this force when the rod makes an angle θ with the vertical.
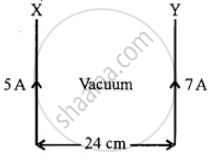
Two infinitely long current carrying conductors X and Y are kept parallel to each other, 24 cm apart in a vacuum. They carry currents of 5A and 7A respectively, in the same direction, as shown in the figure below. Find the position of a neutral point, i.e., a point where resultant magnetic flux density is zero. (Ignore earth’s magnetic field).
A straight horizontal conducting rod of length 0.45 m and mass 60 g is suspended by two vertical wires at its ends. A current of 5.0 A is set up in the rod through the wires.
(a) What magnetic field should be set up normal to the conductor in order that the tension in the wires is zero?
(b) What will be the total tension in the wires if the direction of current is reversed keeping the magnetic field same as before?
(Ignore the mass of the wires) g = 9.8 m s–2.
A conducting loop of resistance R and radius r has its centre at the origin of the coordinate system in a magnetic field of induction B. When it is rotated about y-axis through 90°, the net charge flown in the loop is directly proportional to:

