Advertisements
Advertisements
Question
Bisectors of angles A, B and C of a triangle ABC intersect its circumcircle at D, E and F respectively. Prove that the angles of the triangle DEF are `90^@-1/2A, 90^@-1/2B" and "90^@-1/2C`
Solution

It is given that BE is the bisector of ∠B.
∴ ∠ABE = ∠B/2
However, ∠ADE = ∠ABE (Angles in the same segment for chord AE)
⇒ ∠ADE = ∠B/2
Similarly, ∠ACF = ∠ADF = ∠C/2 (Angle in the same segment for chord AF)
∠D = ∠ADE + ∠ADF
`=(angleB)/2 + (angleC)/2`
`=1/2(angleB+angleC)`
`=1/2(180^@-angleA)`
`=90^@-1/2angleA`
Similarly, it can be proved that
`angleE=90^@-1/2angleB`
`angleF=90^@-1/2angleC`
APPEARS IN
RELATED QUESTIONS
Let the vertex of an angle ABC be located outside a circle and let the sides of the angle intersect equal chords AD and CE with the circle. Prove that ∠ABC is equal to half the difference of the angles subtended by the chords AC and DE at the centre.
ABCD is a parallelogram. The circle through A, B and C intersect CD (produced if necessary) at E. Prove that AE = AD.
In any triangle ABC, if the angle bisector of ∠A and perpendicular bisector of BC intersect, prove that they intersect on the circumcircle of the triangle ABC.
ABCD is a cyclic quadrilateral in BC || AD, ∠ADC = 110° and ∠BAC = 50°. Find ∠DAC.
If the two sides of a pair of opposite sides of a cyclic quadrilateral are equal, prove that its diagonals are equal.
ABCD is a cyclic trapezium with AD || BC. If ∠B = 70°, determine other three angles of the trapezium.
PQRS is a cyclic quadrilateral such that PR is a diameter of the circle. If ∠QPR = 67° and ∠SPR = 72°, then ∠QRS =
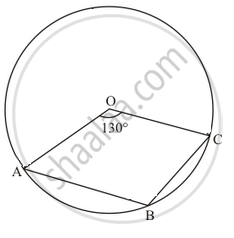
In the given figure, O is the centre of the circle such that ∠AOC = 130°, then ∠ABC =
If a line is drawn parallel to the base of an isosceles triangle to intersect its equal sides, prove that the quadrilateral so formed is cyclic.
If P, Q and R are the mid-points of the sides BC, CA and AB of a triangle and AD is the perpendicular from A on BC, prove that P, Q, R and D are concyclic.
