Advertisements
Advertisements
Question
Find the area of a rhombus if its vertices are (3, 0), (4, 5), (− 1, 4) and (− 2, −1) taken in order.
[Hint: Area of a rhombus = `1/2` (product of its diagonals)]
Solution

Let (3, 0), (4, 5), (−1, 4) and (−2, −1) are the vertices A, B, C, D of a rhombus ABCD.
Length of diagonal AC = `sqrt([3-(-1)]^2 + (0-4)^2)`
= `sqrt((-4)^2 + (4)^2)`
= `sqrt (16 + 16)`
= `4sqrt2`
Length of diagonal BD = `sqrt([4-(-2)]^2+[5-(-1)]^2)`
= `sqrt((-6)^2 + (-6)^2)`
= `sqrt(36+36) `
= `6sqrt2`
Therefore, area of rhombus ABCD = `1/2xx("Product of diagonals")`
= `1/2xx"AC"xx"BD"`
= `1/2xx4sqrt2xx6sqrt2`
= `1/2xx2xx4xx6`
= 24 square units
APPEARS IN
RELATED QUESTIONS
Find the ratio in which the point P(x, 2) divides the line segment joining the points A(12, 5) and B(4, −3). Also, find the value of x.
In what ratio does the x-axis divide the line segment joining the points (2, –3) and (5, 6)? Also, find the coordinates of the point of intersection.
Find the lengths of the medians of a ∆ABC whose vertices are A(7, –3), B(5,3) and C(3,–1)
If A and B are (−2, −2) and (2, −4), respectively, find the coordinates of P such that `"AP" = 3/7 "AB"` and P lies on the line segment AB.
Find the ratio in which P(4, m) divides the line segment joining the points A(2, 3) and B(6, –3). Hence find m.
Find the length of the hypotenuse of a square whose side is 16 cm.
Find the coordinate of a point P which divides the line segment joining :
5(2, 6) and R(9, -8) in the ratio 3: 4.
In what ratio does the point (1, a) divided the join of (−1, 4) and (4, −1) Also, find the value of a.
B is a point on the line segment AC. The coordinates of A and B are (2, 5) and (1, 0). If AC= 3 AB, find the coordinates of C.
Find the ratio in which the point P (2, 4) divides the line joining points (-3, 1) and (7, 6).
The point Q divides segment joining A(3, 5) and B(7, 9) in the ratio 2 : 3. Find the X-coordinate of Q
Find the ratio in which Y-axis divides the point A(3, 5) and point B(– 6, 7). Find the coordinates of the point
The perpendicular bisector of the line segment joining the points A(1, 5) and B(4, 6) cuts the y-axis at ______.
The points A(x1, y1), B(x2, y2) and C(x3, y3) are the vertices of ∆ABC. The median from A meets BC at D. Find the coordinates of the point D.
The fourth vertex D of a parallelogram ABCD, whose three vertices are A(–2, 3), B(6, 7) and C(8, 3), is ______.
In what ratio does the x-axis divide the line segment joining the points (– 4, – 6) and (–1, 7)? Find the coordinates of the point of division.
Complete the following activity to find the coordinates of point P which divides seg AB in the ratio 3:1 where A(4, – 3) and B(8, 5).
Activity: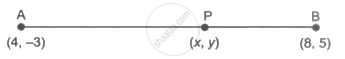
∴ By section formula,
∴ x = `("m"x_2 + "n"x_1)/square`,
∴ x = `(3 xx 8 + 1 xx 4)/(3 + 1)`,
= `(square + 4)/4`,
∴ x = `square`,
∴ y = `square/("m" + "n")`
∴ y = `(3 xx 5 + 1 xx (-3))/(3 + 1)`
= `(square - 3)/4`
∴ y = `square`
Point C divides the line segment whose points are A(4, –6) and B(5, 9) in the ratio 2:1. Find the coordinates of C.
In what ratio does the Y-axis divide the line segment P(– 3, 1) and Q(6, 2)?
Find the co-ordinates of the points of trisection of the line segment joining the points (5, 3) and (4, 5).
