Advertisements
Advertisements
Question
Find the area of each of the following figure:
Solution
In right ΔQRP,
RP2 = PQ2 - QR2
= 152 - 92
= 225 - 81
= 144
⇒ RP = 12cm
Area of ΔQRP
= `(1)/(2) xx "QR" xx "RP"`
= `(1)/(2) xx 9 xx 12`
= 54cm2
∴ Area of given figure
= Area of ΔQRP + Area of ΔRPS
= 54cm2 + 108cm2
= 162cm2.
APPEARS IN
RELATED QUESTIONS
Show that the diagonals of a square are equal and bisect each other at right angles.
Diagonal AC of a parallelogram ABCD bisects ∠A (see the given figure). Show that
- It bisects ∠C also,
- ABCD is a rhombus

State, 'true' or 'false'
Diagonals of a rhombus are equal.
The given figure shows a square ABCD and an equilateral triangle ABP. 
Calculate: (i) ∠AOB
(ii) ∠BPC
(iii) ∠PCD
(iv) Reflex ∠APC
In the figure, AE = BE. Prove that the area of triangle ACE is equal in area to the parallelogram ABCD.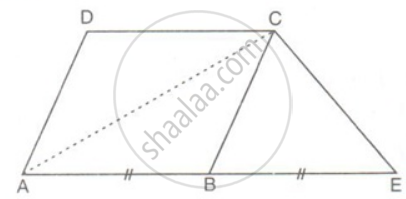
Prove that the median of a triangle divides it into two triangles of equal area.
Find the area of each of the following figure:
Find the area of a parallelogram whose base is 12cm and the height is 5cm.
The area of a floor of a rectangular room is 360m2. If its length is 24cm, find its perimeter.
Find the area of a square whose diagonal is `12sqrt(12)"cm"`
Find the area of a rhombus whose perimeter is 260cm and the length of one of its diagonal is 66cm.
The length and breadth of a rectangular field are in the ratio 8 : 5. A 2m wide path runs all around outside the field. The area of the path is 848m2. Find the length and breadth of the field.
A footpath of uniform width runs all around the inside of a rectangular garden of 40 m x 30 m. If the path occupies 136 m2, find the width of the path.
PQRS is a square with each side 6cm. T is a point on QR such that the `"area of ΔPQT"/"area of trapezium PTRS" = (1)/(3)` Find the length of TR.
In quadrilateral ABCD, ∠A + ∠D = 180º. What special name can be given to this quadrilateral?
All the sides of a parallelogram are of equal length.
Give reason for the following :
Square is also a parallelogram.
Examine whether the following is a polygon. If it is not, say why?
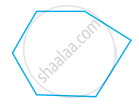
Name polygon.

Make two more examples of this.
