Question

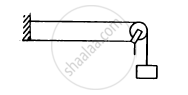
Following figure shows a string stretched by a block going over a pulley. The string vibrates in its tenth harmonic in unison with a particular tuning for. When a beaker containing water is brought under the block so that the block is completely dipped into the beaker, the string vibrates in its eleventh harmonic. Find the density of the material of the block.

Answer 1

Density of the block = ρ
Volume of block = V
∴ Weight of the block is, W = ρVg
∴ Tension in the string, T = W

The tuning fork resonates with different frequencies in the two cases.
Let the tenth harmonic be f₁₀.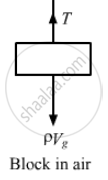

\[f_{11} = \frac{11}{2L}\sqrt{\frac{T'}{m}}\]
\[ = \frac{11}{2L}\sqrt{\frac{\left( \rho - \rho_w \right) Vg}{m}}\]
The frequency (f) of the tuning fork is same.

\[\therefore    f_{10}  =  f_{11} \] 

\[ \Rightarrow \frac{10}{2L}\sqrt{\frac{\rho Vg}{m}} = \frac{11}{2L}\sqrt{\frac{\left( \rho - \rho_\omega \right)  Vg}{m}}\] 

\[ \Rightarrow \frac{100}{121} = \frac{\rho - 1}{\rho}      \left( because  \rho_\omega = 1  gm/cc \right)\] 

\[ \Rightarrow 100  \rho = 121  \rho - 121\] 

\[ \Rightarrow \rho = \frac{121}{21} = 5 . 8  gm/cc\] 

\[= 5 . 8 \times  {10}^3   kg/ m^3\]
Therefore, the required density is \[5 . 8 \times  {10}^3   kg/ m^3\]