Advertisements
Advertisements
Question
Four cows are tethered at the four corners of a square field of side 50 m such that the each can graze the maximum unshared area. What area will be left ungrazed?
Solution
Let r be the radius of the circle.
Thus, we have ;
`r = 50/2 "m"`
= 25 m
Area left ungrazed = (Area of the square) - 4(Area of the sector where r = 25 m and θ = 90°)
`=|(50xx50)-4(3.14xx25xx25xx90/360)|"m"^2`
`=|2500-(4xx(19625)/4)|"m"^2`
`=(2500 - 1962.5) "m"^2`
= 537.5 m2
APPEARS IN
RELATED QUESTIONS
Find the area of sector whose arc length and radius are 10 cm and 5 cm respectively
A chord of a circle of radius 10 cm subtends a right angle at the centre. Find the area of the corresponding:
Major sector [Use π = 3.14]
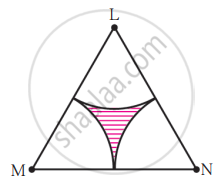
(2) Area of any one of the sectors
The radius of a circle with centre O is 7 cm. Two radii OA and OB are drawn at right angles to each other. Find the areas of minor and major segments.
Prove that the circle drawn with any side of a rhombus as a diameter, passes through the point of intersection of its diagonals.
Find the area of the sector whose arc length and radius are 8 cm and 3 cm respectively.
The area of the largest square that can be inscribed in a circle of radius 12 cm is ____________.
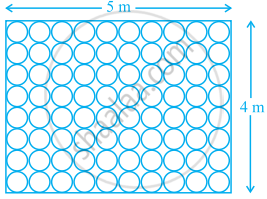
Floor of a room is of dimensions 5 m × 4 m and it is covered with circular tiles of diameters 50 cm each as shown in figure. Find the area of floor that remains uncovered with tiles. (Use π = 3.14)
The length of the arc of a circle of radius 14 cm which subtends an angle of 60° at the centre of the circle is ______.
The area of the sector of a circle of radius 12 cm is 60π cm2. The central angle of this sector is ______.
