Advertisements
Advertisements
Question
Find the area of sector whose arc length and radius are 10 cm and 5 cm respectively
Solution
Length of an arc = 10 cm
Radius (r) = 5 cm
Area of the sector = `r/2xx "length of arc"`
`=5/2xx10`
`=25cm^2`
Thus, the area of the sector is 25 cm2 .
APPEARS IN
RELATED QUESTIONS
Find the area of the sector whose arc length and radius are 14 cm and 6 cm respectively.
Find the area of a sector of a circle with radius 6 cm if angle of the sector is 60° [Use `pi = 22/7`]
A sector of circle of radius 4cm contains an angle of 30°. Find the area of sector
A chord of circle of radius 14cm makes a right angle at the centre. Find the areas of minor and major segments of the circle.
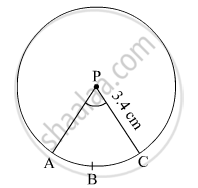
In the given figure, radius of circle is 3.4 cm and perimeter of sector P-ABC is 12.8 cm . Find A(P-ABC).
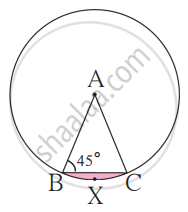
In the given figure, A is the center of the circle. ∠ABC = 45° and AC = 7√2 cm. Find the area of segment BXC.
In the given figure, if O is the centre of the circle, PQ is a chord. \[\angle\] POQ = 90°, area of shaded region is 114 cm2 , find the radius of the circle. \[\pi\] = 3.14)

Choose the correct alternative answer for the following question.
Find the perimeter of a sector of a circle if its measure is 90° and radius is 7 cm.
A cow is tied with a rope of length 14 m at the corner of a rectangular field of dimensions 20 m × 16 m. Find the area of the field in which the cow can graze.
In the given figure, PQ and AB are respectively the arcs of two concentric circles of radii 7 cm and 3.5 cm with centre O. If ∠POQ = 30°, find the area of the shaded region.

Find the area of the shaded region where ABC is a quadrant of radius 5cm and a semicircle is drawn with BC as diameter.

Find the area of a sector of a circle of radius 28 cm and central angle 45°.
Find the area of the minor segment of a circle of radius 14 cm, when the angle of the corresponding sector is 60°.
A piece of wire 20 cm long is bent into the form of an arc of a circle subtending an angle of 60° at its centre. Find the radius of the circle.
Four circular cardboard pieces of radii 7 cm are placed on a paper in such a way that each piece touches other two pieces. Find the area of the portion enclosed between these pieces.
The number of revolutions made by a circular wheel of radius 0.25 m in rolling a distance of 11 km is ______.
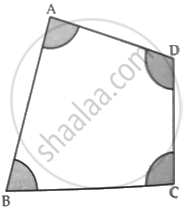
In the given figure, arcs have been drawn of radius 7 cm each with vertices A, B, C and D of quadrilateral ABCD as centres. Find the area of the shaded region.
What is the length of arc of a circle of radius 7 cm which subtends an angle of 90° at the centre of the circle?
