Advertisements
Advertisements
Question
Four cows are tethered at four corners of a square plot of side 50m, so that’ they just cant reach one another. What area will be left ungrazed.
Solution
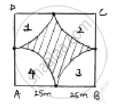
Side of square plot (s) = 50m
Area grazed by four cows is area of sectors represented by 1, 2, 3 and 4.
Radius of each quadrant = 25m = r.
Area of square plot = 𝑠2 = 502 = 2500𝑚2
Area of each quadrant =`1/4 pir^2 =1/4×22/7× 25 × 25 = (625 × 3.14) ×1/4`
Area of ungrazed land = (area of square plot) – 4(area of quadran
=`2500 − 4 (1/4× 3.14 × 625)`
= 2500 – 1962.5 = 537.5 m2
APPEARS IN
RELATED QUESTIONS
In Figure, find the area of the shaded region [Use π = 3.14]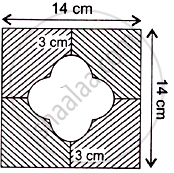
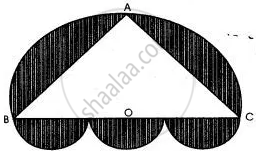
A doorway is decorated as shown in the figure. There are four semi-circles. BC, the diameter of the larger semi-circle is of length 84 cm. Centres of the three equal semicircles lie on BC. ABC is an isosceles triangle with AB = AC. If BO = OC, find the area of the shaded region. (Take `pi = 22/7`)
Find the circumference of a circle whose area is 301.84 cm2.
Find in terms of x the length of the arc that subtends an angle of 30°, at the centre of circle of radius 4 cm.
Below fig shows a sector of a circle, centre O. containing an angle 𝜃°. Prove that Perimeter of shaded region is 𝑟 (tan 𝜃 + sec 𝜃 +`(pitheta)/180`− 1)
Four equal circles, each of radius 5 cm touch each other as shown in fig. Find the area included etween them.
Four equal circles each of radius a, touch each other. Show that area between them is `6/7a^2`
A chord of a circle of radius 30 cm makes an angle of 60° at the centre of the circle. Find the areas of the minor major segments.
A lawn is in the shape of a semi-circle of diameter 42 m. The lawn is surrounded by a flower bed of width 7 m all around. Find the area of the flower bed in m2 .
Find the volume and the surface area of the spheres in the following :
Diameter= 6.3 cm
