Advertisements
Advertisements
Question
The angles of elevation of the top of a tower from two points at a distance of 4 m and 9 m. from the base of the tower and in the same straight line with it are complementary. Prove that the height of the tower is 6 m.
Solution 1
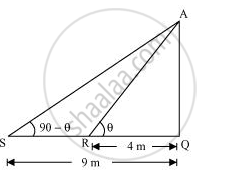
Let AQ be the tower and R, S are the points 4m, 9m away from the base of the tower respectively.
The angles are complementary. Therefore, if one angle is θ, the other will be 90 − θ.
In ΔAQR,
AQ/QR = tanΘ
AQ/4 = tanΘ ... 1
In ΔAQS,
AQ/SQ = tan(90 - Θ)
AQ/9 = cot Θ ...2
On multiplying equations (i) and (ii), we obtain
(AQ/4)(AQ/9) = (tanΘ).(cot Θ)
`(AQ^2)/36 = 1`
`AQ^2 = 36`
`AQ = sqrt36 = +-6`
However, height cannot be negative.
Therefore, the height of the tower is 6 m
Solution 2
Let AB be the tower and C and D be two points such that AC = 4m and AD 9m.
Let:
`AB = hm, ∠BCA=theta and ∠BDA= 90° - theta`
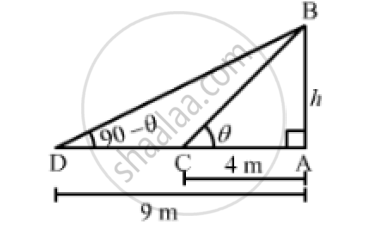
In the right ΔBCA,we have:
`tan theta = (AB)/(AC)`
`⇒ tan theta = h/4 ` ...............(1)
In the right ΔBDA,we have:
` tan (90° - theta ) = (AB) /(AD)`
`⇒ cot theta = h/9 [ tan (90° - theta ) = cot theta]`
`⇒1/ tan theta = h/9 ................(2) [ cot theta = 1/ tan theta]`
Multiplying equations (1) and (2), we get
`tan theta xx 1/ tan theta = h/4xxh/9`
`⇒ 1=( h^2)/36`
`⇒ 36=h^2`
`⇒h = +-6`
Height of a tower cannot be negative
∴Height of the tower = 6 m
RELATED QUESTIONS
A person standing on the bank of river observes that the angle of elevation of the top of a tree standing on the opposite bank is 60°. When he moves 40 m away from the bank, he finds the angle of elevation to be 30°. Find the height of the tree and width of the river. `(sqrt 3=1.73)`
The angles of depression of the top and bottom of a 50 m high building from the top of a tower are 45° and 60° respectively. Find the height of the tower and the horizontal distance between the tower and the building (use `sqrt3`=1.73)
From the top of a building 30 m high, the top and bottom of a tower are observed to have angles of depression 30º and 45º respectively. Find the height of the tower
A man sitting at a height of 20 m on a tall tree on a small island in the middle of a river observes two poles directly opposite to each other on the two banks of the river and in line with the foot of the tree. If the angles of depression of the feet of the poles from a point at which the man is sitting on the tree on either side of the river are 60° and 30°respectively. Find the width of the river.
A man on the deck of a ship is 10 m above the water level. He observes that the angle of elevation of the top of a cliff is 45° and the angle of depression of the base is 300. Calculate the distance of the cliff from the ship and the height of the cliff.
A tower stands vertically on the ground. From a point on the ground which is 25 m away from the foot of the tower, the angle of elevation of the top of the tower is found to be 45°. Then the height (in meters) of the tower is
The angles of elevation and depression of the top and bottom of a lamp post from the top of a 66 m high apartment are 60° and 30° respectively. Find the height of the lamp post
An observer 1.5 m tall is 28.5 m away from a tower. The angle of elevation of the top of the tower from her eyes is 45°. What is the height of the tower?
A contractor plans to install two slides for the children to play in a park. For the children below the age of 5 years, she prefers to have a slide whose top is at a height of 2m and is inclined at an angle of 30° to the ground. What should be the length of the slide?
From the top of a tower h m high, the angles of depression of two objects, which are in line with the foot of the tower are α and β (β > α). Find the distance between the two objects.
