Advertisements
Advertisements
Question
If a chord AB subtends an angle of 60° at the centre of a circle, then angle between the tangents at A and B is also 60°.
Options
True
False
Solution
This statement is False.
Explanation:
Consider the given figure.
In which we have a circle with centre O and AB a chord with ∠AOB = 60°
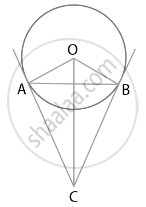
Since, tangent to any point on the circle is perpendicular to the radius through point of contact,
We get,
OA ⊥ AC and OB ⊥ CB
∠OBC = ∠OAC = 90° ...[Equation (1)]
Using angle sum property of quadrilateral in Quadrilateral AOBC,
We get,
∠OBC + ∠OAC + ∠AOB + ∠ACB = 360°
90° + 90° + 60° + ∠ACB = 360°
∠ACB = 120°
Hence, the angle between two tangents is 120°.
APPEARS IN
RELATED QUESTIONS
Two circles touch each other externally at P. AB is a common tangent to the circles touching them at A and B. The value of ∠ L APB is
(A) 30°
(B) 45°
(C) 60°
(D) 90°
In Fig. 1, PA and PB are tangents to the circle with centre O such that ∠APB = 50°. Write the measure of ∠OAB.

A point P is 26 cm away from O of circle and the length PT of the tangent drawn from P to the circle is 10 cm. Find the radius of the circle.
The lengths of three consecutive sides of a quadrilateral circumscribing a circle are 4cm,5cm and 7cm respectively. Determine the length of fourth side.
Two parallel chords are drawn in a circle of diameter 30.0 cm. The length of one chord is 24.0 cm and the distance between the two chords is 21.0 cm; find the length of another chord.
From an external point P , tangents PA = PB are drawn to a circle with centre O . If \[\angle PAB = {50}^o\] , then find \[\angle AOB\]
Draw a circle of radius 6 cm. In the circle, draw a chord AB = 6 cm.
(i) If O is the center of the circle, join OA and OB.
(ii) Assign a special name to ∆AOB
(iii) Write the measure of angle AOB.
If a number of circles touch a given line segment PQ at a point A, then their centres lie on the perpendicular bisector of PQ.
The circumcentre of the triangle ABC is O. Prove that ∠OBC + ∠BAC = 90º.
If radius of a circle is 5 cm, then find the length of longest chord of a circle.
