Advertisements
Advertisements
Question
In a ∆ABC, AD is the bisector of ∠BAC. If AB = 8 cm, BD = 6 cm and DC = 3 cm. Find AC
Options
4 cm
6 cm
3 cm
8 cm
Solution
Given: In a ΔABC, AD is the bisector of angle BAC. AB = 8cm, and DC = 3cm and BD = 6cm.
To find: AC
We know that the internal bisector of angle of a triangle divides the opposite side internally in the ratio of the sides containing the angle.
Hence,
`(AB)/(AC)=(BD)/(DC)`
`8/(AC)=6/3`
`8/(AC)=(8xx3)/6`
`AC= 4cm`
Hence we got the result `a`
APPEARS IN
RELATED QUESTIONS
D and E are points on the sides AB and AC respectively of a ΔABC. In each of the following cases, determine whether DE║BC or not.
AB = 10.8cm, AD = 6.3cm, AC = 9.6cm and EC = 4cm.

In the given figure,

AB || DC prove that
DM × BV = BM ✕ DU
State basic proportionality theorem and its converse.
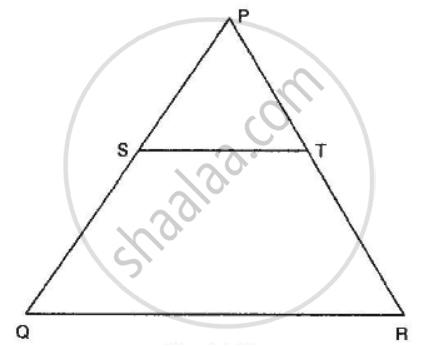
In the given figure, S and T are points on the sides PQ and PR respectively of ∆PQR such that PT = 2 cm, TR = 4 cm and ST is parallel to QR. Find the ratio of the areas of ∆PST and ∆PQR.
The areas of two similar triangles ∆ABC and ∆DEF are 144 cm2 and 81 cm2 respectively. If the longest side of larger ∆ABC be 36 cm, then the longest side of the smaller triangle ∆DEF is
The length of the hypotenuse of an isosceles right triangle whose one side is\[4\sqrt{2} cm\]
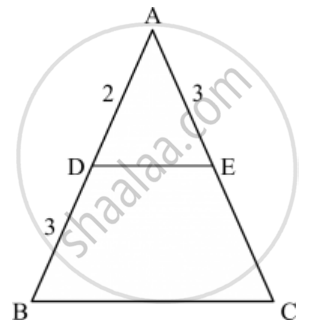
In the given figure, if ∠ADE = ∠ABC, then CE =
A chord of a circle of radius 10 cm subtends a right angle at the centre. The length of the chord (in cm) is
In a ∆ABC, perpendicular AD from A and BC meets BC at D. If BD = 8 cm, DC = 2 cm and AD = 4 cm, then
∆ABC is a right triangle right-angled at A and AD ⊥ BC. Then, \[\frac{BD}{DC} =\]
