Advertisements
Advertisements
Question
In the following figure, write BC, AC, and CD in ascending order of their lengths.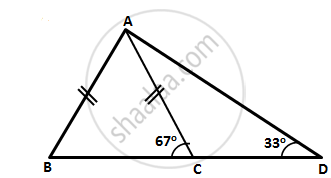
Solution

In ΔABC,
AB = AC
⇒ ∠ABC = ∠ACB ..(angles opposite to equal sides are equal)
⇒ ∠ABC = ∠ACB = 67°
⇒ ∠BAC = 180° - ∠ABC - ∠ACB ...(Angle sum property)
⇒ ∠BAC = 180° - 67° - 67° = 46°
Since ∠BAC < ∠ABC, we have
BC < AC ...(1)
Now, ∠ACD = 180° - ACB ...(Linear pair)
⇒ ∠ACD = 180° - 67° = 113°
Thus, in ΔACD,
∠CAD = 180°- ∠ACD - ∠ADC
⇒ ∠CAD = 180° - 113° - 33° = 34°
Since ∠ADC < ∠CAD, we have
AC < CD ...(2)
From (1) and (2), we have
BC < AC < CD.
APPEARS IN
RELATED QUESTIONS
In the given figure, PR > PQ and PS bisects ∠QPR. Prove that ∠PSR >∠PSQ.
In a huge park people are concentrated at three points (see the given figure):

A: where there are different slides and swings for children,
B: near which a man-made lake is situated,
C: which is near to a large parking and exit.
Where should an ice-cream parlour be set up so that maximum number of persons can approach it?
(Hint: The parlor should be equidistant from A, B and C)
Complete the hexagonal and star shaped rangolies (see the given figures) by filling them with as many equilateral triangles of side 1 cm as you can. Count the number of triangles in each case. Which has more triangles?
Name the greatest and the smallest sides in the following triangles:
ΔDEF, ∠D = 32°, ∠E = 56° and ∠F = 92°.
Arrange the sides of the following triangles in an ascending order:
ΔDEF, ∠D = 38°, ∠E = 58°.
Prove that the hypotenuse is the longest side in a right-angled triangle.
For any quadrilateral, prove that its perimeter is greater than the sum of its diagonals.
In ΔPQR, PR > PQ and T is a point on PR such that PT = PQ. Prove that QR > TR.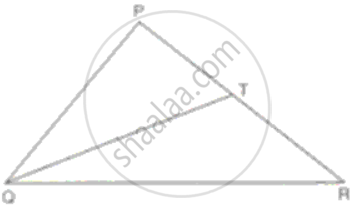
ABCD is a trapezium. Prove that:
CD + DA + AB + BC > 2AC.
Prove that in an isosceles triangle any of its equal sides is greater than the straight line joining the vertex to any point on the base of the triangle.
