Advertisements
Advertisements
Question
In fig.. O is the center of the circle and BCD is tangent to it at C. Prove that ∠BAC +
∠ACD = 90°
Solution
Given
O is center of circle
BCD is tangent.
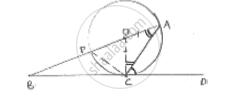
Required to prove: ∠BAC + ∠ACD = 90°
Proof: OA = OC [radius]
In ΔOAC, angles opposite to equal sides are equal.
∠OAC = ∠OCA …. (i)
∠OCD = 90° [tangent is radius are perpendicular at point of contact]
∠ACD + ∠OCA = 90°
∠ACD + ∠OAC = 90° [∵ ∠OAC = ∠BAC]
∠ACD + ∠BAC = 90° ⟶ Hence proved
APPEARS IN
RELATED QUESTIONS
Draw different pairs of circles. How many points does each pair have in common? What is the maximum number of common points?
From an external point P, tangents PA and PB are drawn to a circle with center O. If CD is the tangent to the circle at a point E and PA = 14cm, find the perimeter of ΔPCD.

Two circles touch internally. The sum of their areas is 116 π cm2 and the distance between their centres is 6 cm. Find the radii of the circles ?
In a cyclic quadrilateral ABCD if AB || CD and ∠B = 70°, find the remaining angles.
In the given figure, ABC is a right triangle right-angled at B such that BC = 6 cm and AB = 8 cm. Find the radius of its incircle.

Find the length of the chord of a circle in the following when:
Radius is 1. 7cm and the distance from the centre is 1.5 cm
The chord of length 30 cm is drawn at the distance of 8 cm from the centre of the circle. Find the radius of the circle
In the figure, O is the center of the circle. Line AQ is a tangent. If OP = 3, m(arc PM) = 120°, then find the length of AP.
The length of tangent from an external point on a circle is always greater than the radius of the circle.
In the following figure, if AOB is a diameter of the circle and AC = BC, then ∠CAB is equal to ______.

